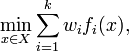Scalarizing Multiobjective Optimization Problems
Scalarizing a multiobjective optimization problem means formulating a single-objective optimization problems such that optimal solutions to the single-objective optimization problem are Pareto optimal solutions to the multiobjective optimization problem. With different parameters for the scalarization, different Pareto optimal solutions are produced. A well-known example is the so-called linear scalarization (see, e.g.,)
where the weights of the objectives are the parameters of the scalarization.
Read more about this topic: Multi-objective Optimization
Famous quotes containing the word problems:
“If combat means living in a ditch, females have biological problems staying in a ditch for 30 days because they get infections.... Males are biologically driven to go out and hunt giraffes.”
—Newt Gingrich (b. 1943)