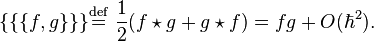Moyal Bracket - Sine Backet and Cosine Bracket

Sine Backet and Cosine Bracket

Next to the sine bracket discussed, Groenewold further introduced the cosine bracket, elaborated by Baker,:

Here, again, ★ is the star-product operator in phase space, f and g are differentiable phase-space functions, and f g is the ordinary product.

The sine and cosine brackets are, respectively, the results of antisymmetrizing and symmetrizing the star product. Thus, as the sine bracket is the Wigner map of the commutator, the cosine bracket is the Wigner image of the anticommutator in standard quantum mechanics. Similarly, as the Moyal bracket equals the Poisson bracket up to higher orders of ħ, the cosine bracket equals the ordinary product up to higher orders of ħ. In the classical limit, the Moyal bracket helps reduction to the Liouville equation (formulated in terms of the Poisson bracket), as the cosine bracket leads to the classical Hamilton–Jacobi equation.

The sine and cosine bracket also stand in relation to equations of a purely algebraic description of quantum mechanics.