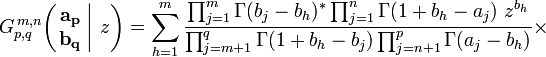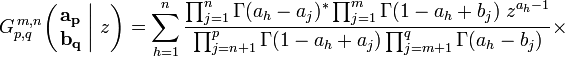Meijer G-function - Relationship Between The G-function and The Generalized Hypergeometric Function | Relationship G-function Generalized Hypergeometric Function

Relationship Between The G-function and The Generalized Hypergeometric Function

If the integral converges when evaluated along the second path introduced above, and if no confluent poles appear among the Γ(b_j − s), j = 1, 2, ..., m, then the Meijer G-function can be expressed as a sum of residues in terms of generalized hypergeometric functions _pF_q−1 (Slater's theorem):

$G_{p,q}^{\,m,n} \!\left( \left. \begin{matrix} \mathbf{a_p} \\ \mathbf{b_q} \end{matrix} \; \right| \, z \right) = \sum_{h=1}^m \frac{\prod_{j=1}^m \Gamma(b_j - b_h)^* \prod_{j=1}^n \Gamma(1+b_h - a_j) \; z^{b_h}} {\prod_{j=m+1}^q \Gamma(1+b_h - b_j) \prod_{j=n+1}^p \Gamma(a_j - b_h)} \times$

$\times \; _{p}F_{q-1} \!\left( \left. \begin{matrix} 1+b_h - \mathbf{a_p} \\ (1+b_h - \mathbf{b_q})^* \end{matrix} \; \right| \, (-1)^{p-m-n} \; z \right) .$

For the integral to converge along the second path one must have either p < q, or p = q and |z| < 1, and for the poles to be distinct no pair among the b_j, j = 1, 2, ..., m, may differ by an integer or zero. The asterisks in the relation remind us to ignore the contribution with index j = h as follows: In the product this amounts to replacing Γ(0) with 1, and in the argument of the hypergeometric function, if we recall the meaning of the vector notation,

$1 + b_h - \mathbf{b_q} = (1 + b_h - b_1), \,\dots, \,(1 + b_h - b_j), \,\dots, \,(1 + b_h - b_q),$

this amounts to shortening the vector length from q to q−1.

Note that when m = 0, the second path does not contain any pole, and so the integral must vanish identically,

$G_{p,q}^{\,0,n} \!\left( \left. \begin{matrix} \mathbf{a_p} \\ \mathbf{b_q} \end{matrix} \; \right| \, z \right) = 0,$

if either p < q, or p = q and |z| < 1.

Similarly, if the integral converges when evaluated along the third path above, and if no confluent poles appear among the Γ(1 − a_k + s), k = 1, 2, ..., n, then the G-function can be expressed as:

$G_{p,q}^{\,m,n} \!\left( \left. \begin{matrix} \mathbf{a_p} \\ \mathbf{b_q} \end{matrix} \; \right| \, z \right) = \sum_{h=1}^n \frac{\prod_{j=1}^n \Gamma(a_h - a_j)^* \prod_{j=1}^m \Gamma(1-a_h + b_j) \; z^{a_h-1}} {\prod_{j=n+1}^p \Gamma(1-a_h + a_j) \prod_{j=m+1}^q \Gamma(a_h - b_j)} \times$

$\times \; _{q}F_{p-1} \!\left( \left. \begin{matrix} 1-a_h + \mathbf{b_q} \\ (1-a_h + \mathbf{a_p})^* \end{matrix} \; \right| \, (-1)^{q-m-n} z^{-1} \right) .$

For this, either p > q, or p = q and |z| > 1 are required, and no pair among the a_k, k = 1, 2, ..., n, may differ by an integer or zero. For n = 0 one consequently has:

$G_{p,q}^{\,m,0} \!\left( \left. \begin{matrix} \mathbf{a_p} \\ \mathbf{b_q} \end{matrix} \; \right| \, z \right) = 0,$

if either p > q, or p = q and |z| > 1.

On the other hand, any generalized hypergeometric function can readily be expressed in terms of the Meijer G-function:

$\; _{p}F_{q} \!\left( \left. \begin{matrix} \mathbf{a_p} \\ \mathbf{b_q} \end{matrix} \; \right| \, z \right) = \frac {\Gamma(\mathbf{b_q})} {\Gamma(\mathbf{a_p})} \; G_{p,\,q+1}^{\,1,\,p} \!\left( \left. \begin{matrix} 1-\mathbf{a_p} \\ 0,1 - \mathbf{b_q} \end{matrix} \; \right| \, -z \right) = \frac {\Gamma(\mathbf{b_q})} {\Gamma(\mathbf{a_p})} \; G_{q+1,\,p}^{\,p,\,1} \!\left( \left. \begin{matrix} 1,\mathbf{b_q} \\ \mathbf{a_p} \end{matrix} \; \right| \, -z^{-1} \right),$

where we have made use of the vector notation:

$\Gamma(\mathbf{a_p}) = \prod_{j=1}^p \Gamma(a_j).$

This holds unless a nonpositive integer value of at least one of its parameters a_p reduces the hypergeometric function to a finite polynomial, in which case the gamma prefactor of either G-function vanishes and the parameter sets of the G-functions violate the requirement a_k − b_j ≠ 1, 2, 3, ... for k = 1, 2, ..., n and j = 1, 2, ..., m from the definition above. Apart from this restriction, the relationship is valid whenever the generalized hypergeometric series _pF_q(z) converges, i. e. for any finite z when p ≤ q, and for |z| < 1 when p = q + 1. In the latter case, the relation with the G-function automatically provides the analytic continuation of _pF_q(z) to |z| ≥ 1 with a branch cut from 1 to ∞ along the real axis. Finally, the relation furnishes a natural extension of the definition of the hypergeometric function to orders p > q + 1. By means of the G-function we can thus solve the generalized hypergeometric differential equation for p > q + 1 as well.

Read more about this topic: Meijer G-function

Famous quotes containing the words relationship between the, relationship between, relationship, generalized and/or function:

“We must introduce a new balance in the relationship between the individual and the government—a balance that favors greater individual freedom and self-reliance.”
—Gerald R. Ford (b. 1913)

“Living in cities is an art, and we need the vocabulary of art, of style, to describe the peculiar relationship between man and material that exists in the continual creative play of urban living. The city as we imagine it, then, soft city of illusion, myth, aspiration, and nightmare, is as real, maybe more real, than the hard city one can locate on maps in statistics, in monographs on urban sociology and demography and architecture.”
—Jonathan Raban (b. 1942)

“Every man is in a state of conflict, owing to his attempt to reconcile himself and his relationship with life to his conception of harmony. This conflict makes his soul a battlefield, where the forces that wish this reconciliation fight those that do not and reject the alternative solutions they offer. Works of art are attempts to fight out this conflict in the imaginative world.”
—Rebecca West (1892–1983)

“One is conscious of no brave and noble earnestness in it, of no generalized passion for intellectual and spiritual adventure, of no organized determination to think things out. What is there is a highly self-conscious and insipid correctness, a bloodless respectability submergence of matter in manner—in brief, what is there is the feeble, uninspiring quality of German painting and English music.”
—H.L. (Henry Lewis)

“Any translation which intends to perform a transmitting function cannot transmit anything but information—hence, something inessential. This is the hallmark of bad translations.”
—Walter Benjamin (1892–1940)