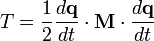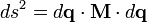Maupertuis' Principle - Jacobi's Formulation

Jacobi's Formulation

For many systems, the kinetic energy is quadratic in the generalized velocities

T = \frac{1}{2} \frac{d\mathbf{q}}{dt} \cdot \mathbf{M} \cdot \frac{d\mathbf{q}}{dt}

although the mass tensor may be a complicated function of the generalized coordinates . For such systems, a simple relation relates the kinetic energy, the generalized momenta and the generalized velocities

2 T = \mathbf{p} \cdot \dot{\mathbf{q}}

provided that the potential energy does not involve the generalized velocities. By defining a normalized distance or metric in the space of generalized coordinates

ds^{2} = d\mathbf{q} \cdot \mathbf{M} \cdot d\mathbf{q}

one may immediately recognize the mass tensor as a metric tensor. The kinetic energy may be written in a massless form

T = \frac{1}{2} \left( \frac{ds}{dt} \right)^{2}

or, equivalently,

2 T dt = \mathbf{p} \cdot d\mathbf{q} = \sqrt{2 T} \ ds.

Hence, the abbreviated action can be written

\mathcal{S}_{0} \ \stackrel{\mathrm{def}}{=}\ \int \mathbf{p} \cdot d\mathbf{q} = \int ds \sqrt{2}\sqrt{E_{tot} - V(\mathbf{q})}

since the kinetic energy equals the (constant) total energy minus the potential energy . In particular, if the potential energy is a constant, then Jacobi's principle reduces to minimizing the path length in the space of the generalized coordinates, which is equivalent to Hertz's principle of least curvature.

Read more about this topic:  Maupertuis' Principle

Famous quotes containing the words jacobi and/or formulation:

    “... [the] special relation of women to children, in which the heart of the world has always felt there was something sacred, serves to impress upon women certain tendencies, to endow them with certain virtues ... which will render them of special value in public affairs.”
    —Mary Putnam Jacobi (1842–1906)

    “Art is an experience, not the formulation of a problem.”
    —Lindsay Anderson (b. 1923)