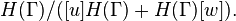Massey Triple Product
The Massey product is defined algebraically at the level of chains (at the level of a differential graded algebra, or DGA); the Massey product of elements of cohomology is obtained by lifting the elements to equivalence classes of chains, taking the Massey products of these, and then pushing down to cohomology. This may result in a well-defined cohomology class, or may result in indeterminacy.
In a DGA Γ with differential d, the cohomology H(Γ) is an algebra. Define to be (-1)deg(u)+1u. The cohomology class of an element u of Γ will be denoted by . The Massey triple product of three cohomology classes is defined by
The Massey product of 3 cohomology classes is not an element of H(Γ) but a set of elements of H(Γ), possibly empty and possibly containing more than one element. If have degrees then the Massey product has degree with the −1 coming from the differential 'd.
The Massey product is nonempty if the products uv and vw are both exact, in which case all its elements are in the same element of the quotient group
So the Massey product can be regarded as a function defined on triples of classes such that the product of the first or last two is zero, taking values in the above quotient group.
More casually, if the two pairwise products and both vanish in homology, i.e. and for some chains and, then the triple product vanishes "for two different reasons" – it is the boundary of and (since and because elements of homology are cycles). The bounding chains and have indeterminacy, which disappears when one moves to homology, and since and have the same boundary, subtracting them (the sign convention is to correctly handle the grading) gives a cocycle (the boundary of the difference vanishes), and one thus obtains a well-defined element of cohomology – this step is analogous to defining the n+1st homotopy or homology group in terms of indeterminacy in null-homotopies/null-homologies of n-dimensional maps/chains.
Geometrically, in singular cohomology of a manifold, one can interpret the product dually in terms of bounding manifolds and intersections, following Poincaré duality: dual to cocycles are cycles, often representable as closed manifolds (without boundary), dual to product is intersection, and dual to the subtraction of the bounding products is gluing the two bounding manifolds together along the boundary, obtaining a closed manifold which represents the homology class dual of the Massey product. In reality homology classes of manifolds cannot always be represented by manifolds – a representing cycle may have singularities – but with this caveat the dual picture is correct.
Read more about this topic: Massey Product
Famous quotes containing the words triple and/or product:
“The triple pillar of the world transformed
Into a strumpet’s fool.”
—William Shakespeare (1564–1616)
“Perhaps I am still very much of an American. That is to say, naïve, optimistic, gullible.... In the eyes of a European, what am I but an American to the core, an American who exposes his Americanism like a sore. Like it or not, I am a product of this land of plenty, a believer in superabundance, a believer in miracles.”
—Henry Miller (1891–1980)