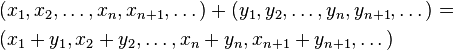L^p Space - The p-norm in Countably Infinite Dimensions

The p-norm in Countably Infinite Dimensions

For more details on this topic, see Sequence space.

The p-norm can be extended to vectors that have an infinite number of components, which yields the space . This contains as special cases:

• , the space of sequences whose series is absolutely convergent,
• , the space of square-summable sequences, which is a Hilbert space, and
• , the space of bounded sequences.

The space of sequences has a natural vector space structure by applying addition and scalar multiplication coordinate by coordinate. Explicitly, for an infinite sequence of real (or complex) numbers, define the vector sum to be

while the scalar action is given by

Define the p-norm

Here, a complication arises, namely that the series on the right is not always convergent, so for example, the sequence made up of only ones, (1, 1, 1, …), will have an infinite p-norm (length) for every finite p ≥ 1. The space ℓp is then defined as the set of all infinite sequences of real (or complex) numbers such that the p-norm is finite.

One can check that as p increases, the set ℓp grows larger. For example, the sequence

is not in ℓ1, but it is in ℓp for p > 1, as the series

diverges for p = 1 (the harmonic series), but is convergent for p > 1.

One also defines the ∞-norm using the supremum:

and the corresponding space ℓ∞ of all bounded sequences. It turns out that see

if the right-hand side is finite, or the left-hand side is infinite. Thus, we will consider ℓp spaces for 1 ≤ p ≤ ∞.

The p-norm thus defined on ℓp is indeed a norm, and ℓp together with this norm is a Banach space. The fully general Lp space is obtained — as seen below — by considering vectors, not only with finitely or countably-infinitely many components, but with "arbitrarily many components"; in other words, functions. An integral instead of a sum is used to define the p-norm.