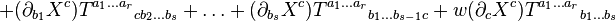Lie Derivative - Coordinate Expressions

Coordinate Expressions

In local coordinate notation, for a type (r,s) tensor field, the Lie derivative along is

\begin{align} (\mathcal{L}_X T) ^{a_1 \ldots a_r}{}_{b_1 \ldots b_s} = & X^c(\partial_c T^{a_1 \ldots a_r}{}_{b_1 \ldots b_s}) \\ & - (\partial_c X ^{a_1}) T ^{c a_2 \ldots a_r}{}_{b_1 \ldots b_s} - \ldots - (\partial_c X^{a_r}) T ^{a_1 \ldots a_{r-1}c}{}_{b_1 \ldots b_s} \\ & + (\partial_{b_1} X^c) T ^{a_1 \ldots a_r}{}_{c b_2 \ldots b_s} + \ldots + (\partial_{b_s}X^c) T ^{a_1 \ldots a_r}{}_{b_1 \ldots b_{s-1} c} \end{align}

here, the notation means taking the partial derivative with respect to the coordinate . Alternatively, if we are using a torsion-free connection (e.g. the Levi Civita connection), then the partial derivative can be replaced with the covariant derivative . The Lie derivative of a tensor is another tensor of the same type, i.e. even though the individual terms in the expression depend on the choice of coordinate system, the expression as a whole results in a tensor

which is independent of any coordinate system.

The definition can be extended further to tensor densities of weight w for any real w. If T is such a tensor density, then its Lie derivative is a tensor density of the same type and weight.

+ (\partial_{b_1} X^c) T ^{a_1 \ldots a_r}{}_{c b_2 \ldots b_s} + \ldots + (\partial_{b_s} X^c) T ^{a_1 \ldots a_r}{}_{b_1 \ldots b_{s-1} c} + w (\partial_{c} X^c) T ^{a_1 \ldots a_r}{}_{b_1 \ldots b_{s}}

Notice the new term at the end of the expression.

Read more about this topic:  Lie Derivative

Famous quotes containing the word expressions:

    “We ought to celebrate this hour by expressions of manly joy. Not thanks, not prayer seem quite the highest or truest name for our communication with the infinite,—but glad and conspiring reception,—reception that becomes giving in its turn, as the receiver is only the All-Giver in part and infancy.”
    —Ralph Waldo Emerson (1803–1882)