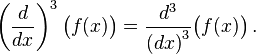Leibniz's Notation - Leibniz's Notation For Differentiation

Leibniz's Notation For Differentiation

In Leibniz's notation for differentiation, the derivative of the function f(x) is written:

If we have a variable representing a function, for example if we set

then we can write the derivative as:

Using Lagrange's notation, we can write:

Using Newton's notation, we can write:

For higher derivatives, we express them as follows:

denotes the nth derivative of ƒ(x) or y respectively. Historically, this came from the fact that, for example, the third derivative is:

which we can loosely write as:

Now drop the parentheses and we have:

The chain rule and integration by substitution rules are especially easy to express here, because the "d" terms cancel:

etc., and: