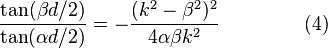Lamb Waves - Lamb's Characteristic Equations

Lamb's Characteristic Equations

In general, elastic waves in solid materials are guided by the boundaries of the media in which they propagate. An approach to guided wave propagation, widely used in physical acoustics, is to seek sinusoidal solutions to the wave equation for linear elastic waves subject to boundary conditions representing the structural geometry. This is a classic eigenvalue problem.

Waves in plates were among the first guided waves to be analyzed in this way. The analysis was developed and published in 1917 by Horace Lamb, a leader in the mathematical physics of his day.

Lamb's equations were derived by setting up formalism for a solid plate having infinite extent in the x and y directions, and thickness d in the z direction. Sinusoidal solutions to the wave equation were postulated, having x- and z-displacements of the form

This form represents sinusoidal waves propagating in the x direction with wavelength 2π/k and frequency ω/2π. Displacement is a function of x, z, t only; there is no displacement in the y direction and no variation of any physical quantities in the y direction.

The physical boundary condition for the free surfaces of the plate is that the component of stress in the z direction at z = +/- d/2 is zero. Applying these two conditions to the above-formalized solutions to the wave equation, a pair of characteristic equations can be found. These are:

and

where

Inherent in these equations is a relationship between the angular frequency ω and the wave number k. Numerical methods are used to find the phase velocity c_p = fλ = ω/k, and the group velocity c_g = dω/dk, as functions of d/λ or fd. c_l and c_t are the longitudinal wave and shear wave velocities respectively.

The solution of these equations also reveals the precise form of the particle motion, which equations (1) and (2) represent in generic form only. It is found that equation (3) gives rise to a family of waves whose motion is symmetrical about the midplane of the plate (the plane z = 0), while equation (4) gives rise to a family of waves whose motion is antisymmetric about the midplane. Figure 1 illustrates a member of each family.

Lamb’s characteristic equations were established for waves propagating in an infinite plate - a homogeneous, isotropic solid bounded by two parallel planes beyond which no wave energy can propagate. In formulating his problem, Lamb confined the components of particle motion to the direction of the plate normal (z-direction) and the direction of wave propagation (x-direction). By definition, Lamb waves have no particle motion in the y-direction. Motion in the y-direction in plates is found in the so-called SH or shear-horizontal wave modes. These have no motion in the x- or z-directions, and are thus complementary to the Lamb wave modes. These two are the only wave types which can propagate with straight, infinite wave fronts in a plate as defined above.