Khinchin's Constant

Khinchin's Constant

In number theory, Aleksandr Yakovlevich Khinchin proved that for almost all real numbers x, coefficients ai of the continued fraction expansion of x have a finite geometric mean that is independent of the value of x and is known as Khinchin's constant.

That is, for

it is almost always true that

\lim_{n \rightarrow \infty } \left( \prod_{i=1}^n a_i \right) ^{1/n} = K_0

where is Khinchin's constant

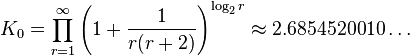K_0 = \prod_{r=1}^\infty {\left( 1+{1\over r(r+2)}\right)}^{\log_2 r} \approx 2.6854520010\dots (sequence A002210 in OEIS).

Among the numbers x whose continued fraction expansions do not have this property are rational numbers, solutions of quadratic equations with rational coefficients (including the golden ratio Φ), and the base of the natural logarithm e.

Khinchin is sometimes spelled Khintchine (the French transliteration) in older mathematical literature.

Read more about Khinchin's Constant:  Sketch of Proof, Series Expressions, Hölder Means, Harmonic Mean, Open Problems

Famous quotes containing the word constant:

    “The thirsty earth soaks up the rain,
    And drinks, and gapes for drink again.
    The plants suck in the earth, and are
    With constant drinking fresh and fair.”
    —Abraham Cowley (1618–1667)