Two-dimensional J-integral
The two-dimensional J-integral was originally defined as (see Figure 1 for an illustration)
where is the strain energy density, are the coordinate directions, is the surface traction vector, is the normal to the curve, is the Cauchy stress tensor, and is the displacement vector. The strain energy density is given by
The J-Integral around a crack tip is frequently expressed in a more general form (and in index notation) as
where is the component of the J-integral for crack opening in the direction and is a small region around the crack tip. Using Green's theorem we can show that this integral is zero when the boundary is closed and encloses a region that contains no singularities and is simply connected. If the faces of the crack do not have any surface tractions on them then the J-integral is also path independent.
Rice also showed that the value of the J-integral represents the energy release rate for planar crack growth. The J-integral was developed because of the difficulties involved in computing the stress close to a crack in a nonlinear elastic or elastic-plastic material. Rice showed that if monotonic loading was assumed (without any plastic unloading) then the J-integral could be used to compute the energy release rate of plastic materials too.
-
Proof that the J-integral is zero over a closed path To show the path independence of the J-integral, we first have to show that the value of is zero over a closed contour in a simply connected domain. Let us just consider the expression for which is We can write this as
From Green's theorem (or the two-dimensional divergence theorem) we have
Using this result we can express as
where is the area enclosed by the contour . Now, if there are no body forces present, equilibrium (conservation of linear momentum) requires that
Also,
Therefore,
From the balance of angular momentum we have . Hence,
The J-integral may then be written as
Now, for an elastic material the stress can be derived from the stored energy function using
Then, using the chain rule of differentiation,
Therefore we have for a closed contour enclosing a simply connected region without any stress singularities.
-
Proof that the J-integral is path-independent Consider the contour . Since this contour is closed and encloses a simply connected region, the J-integral around the contour is zero, i.e.
assuming that counterclockwise integrals around the crack tip have positive sign. Now, since the crack surfaces are parallel to the axis, the normal component on these surfaces. Also, since the crack surfaces are traction free, . Therefore,
Therefore,
and the J-integral is path independent.
Read more about this topic: J Integral






![\begin{align} J_1 & = \int_{A} \cfrac{\partial}{\partial x_j}\left(W \delta_{1j} - \sigma_{jk}~\cfrac{\partial u_k}{\partial x_1}\right) dA \\ & = \int_A \left[\cfrac{\partial W}{\partial x_1} - \cfrac{\partial\sigma_{jk}}{\partial x_j}~\cfrac{\partial u_k}{\partial x_1} - \sigma_{jk}~\cfrac{\partial^2 u_k}{\partial x_1 \partial x_j}\right]~dA \end{align}](http://upload.wikimedia.org/math/8/f/d/8fd53a298c780eb6d70753c2cd9d9b7c.png)

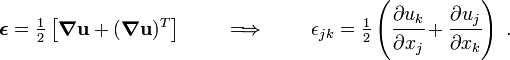


![J_1 = \int_A \left[\cfrac{\partial W}{\partial x_1} - \sigma_{jk}~\cfrac{\partial\epsilon_{jk}}{\partial x_1}\right]~dA](http://upload.wikimedia.org/math/2/2/3/2238db88f38f08ac9129731f880dc12f.png)




