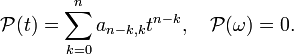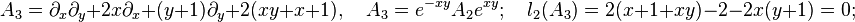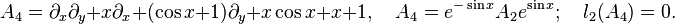Invariant Factorization of LPDOs - Invariant Formulation

Invariant Formulation

Definition The operators, are called equivalent if there is a gauge transformation that takes one to the other:

\tilde{\mathcal{A}} g= e^{-\varphi}\mathcal{A} (e^{\varphi}g).

BK-factorization is then pure algebraic procedure which allows to construct explicitly a factorization of an arbitrary order LPDO in the form

\mathcal{A}=\sum_{j+k\le n}a_{jk}\partial_x^j\partial_y^k=\mathcal{L}\circ \sum_{j+k\le (n-1)}p_{jk}\partial_x^j\partial_y^k

with first-order operator where is an arbitrary simple root of the characteristic polynomial

\mathcal{P}(t)=\sum^n_{k=0}a_{n-k,k}t^{n-k}, \quad \mathcal{P}(\omega)=0.

Factorization is possible then for each simple root iff

for

for

for

and so on. All functions l_2, l_3, l_{31}, l_4, l_{41}, \ \ l_{42}, ... are known functions, for instance,

and so on.

Theorem All functions

l_2= a_{00} - \mathcal{L}(p_6)+p_3p_6, l_3= a_{00} - \mathcal{L}(p_9)+p_3p_9, l_{31}, ....

are invariants under gauge transformations.

Definition Invariants l_2= a_{00} - \mathcal{L}(p_6)+p_3p_6, l_3= a_{00} - \mathcal{L}(p_9)+p_3p_9, l_{31}, .... . are called generalized invariants of a bivariate operator of arbitrary order.

In particular case of the bivariate hyperbolic operator its generalized invariants coincide with Laplace invariants (see Laplace invariant).

Corollary If an operator is factorizable, then all operators equivalent to it, are also factorizable.

Equivalent operators are easy to compute:

e^{-\varphi} \partial_x e^{\varphi}= \partial_x+\varphi_x, \quad e^{-\varphi}\partial_y e^{\varphi}= \partial_y+\varphi_y,
e^{-\varphi} \partial_x \partial_y e^{\varphi}= e^{-\varphi} \partial_x e^{\varphi} e^{-\varphi} \partial_y e^{\varphi}=(\partial_x+\varphi_x) \circ (\partial_y+\varphi_y)

and so on. Some example are given below:

A_1=\partial_x \partial_y + x\partial_x + 1= \partial_x(\partial_y+x), \quad l_2(A_1)=1-1-0=0;
A_2=\partial_x \partial_y + x\partial_x + \partial_y +x + 1, \quad A_2=e^{-x}A_1e^{x};\quad l_2(A_2)=(x+1)-1-x=0;
A_3=\partial_x \partial_y + 2x\partial_x + (y+1)\partial_y +2(xy +x+1), \quad A_3=e^{-xy}A_2e^{xy}; \quad l_2(A_3)=2(x+1+xy)-2-2x(y+1)=0;
A_4=\partial_x \partial_y +x\partial_x + (\cos x +1) \partial_y + x \cos x +x +1, \quad A_4=e^{-\sin x}A_2e^{\sin x}; \quad l_2(A_4)=0.

Read more about this topic:  Invariant Factorization Of LPDOs

Famous quotes containing the word formulation:

    “Art is an experience, not the formulation of a problem.”
    —Lindsay Anderson (b. 1923)