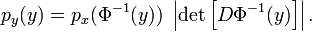Integration By Substitution - Application in Probability

Application in Probability

Substitution can be used to answer the following important question in probability: given a random variable with probability density and another random variable related to by the equation, what is the probability density for ?

It is easiest to answer this question by first answering a slightly different question: what is the probability that takes a value in some particular subset ? Denote this probability . Of course, if has probability density then the answer is

but this isn't really useful because we don't know p_y; it's what we're trying to find in the first place. We can make progress by considering the problem in the variable . takes a value in S whenever X takes a value in, so

Changing from variable x to y gives

$P(Y \in S) = \int_{\Phi^{-1}(S)} p_x(x)~dx = \int_S p_x(\Phi^{-1}(y)) ~ \left|\frac{d\Phi^{-1}}{dy}\right|~dy.$

Combining this with our first equation gives

$\int_S p_y(y)~dy = \int_S p_x(\Phi^{-1}(y)) ~ \left|\frac{d\Phi^{-1}}{dy}\right|~dy$

$p_y(y) = p_x(\Phi^{-1}(y)) ~ \left|\frac{d\Phi^{-1}}{dy}\right|.$

In the case where and depend on several uncorrelated variables, i.e., and, can be found by substitution in several variables discussed above. The result is

$p_y(y) = p_x(\Phi^{-1}(y)) ~ \left|\det \left \right|.$

Read more about this topic: Integration By Substitution

Famous quotes containing the words application and/or probability:

“The human mind is capable of excitement without the application of gross and violent stimulants; and he must have a very faint perception of its beauty and dignity who does not know this.”
—William Wordsworth (1770–1850)

“The source of Pyrrhonism comes from failing to distinguish between a demonstration, a proof and a probability. A demonstration supposes that the contradictory idea is impossible; a proof of fact is where all the reasons lead to belief, without there being any pretext for doubt; a probability is where the reasons for belief are stronger than those for doubting.”
—Andrew Michael Ramsay (1686–1743)