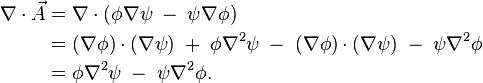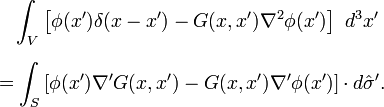Green's Function - Green's Functions For The Laplacian

Green's Functions For The Laplacian

Green's functions for linear differential operators involving the Laplacian may be readily put to use using the second of Green's identities.

To derive Green's theorem, begin with the divergence theorem (otherwise known as Gauss's theorem):

Let and substitute into Gauss' law. Compute and apply the chain rule for the operator:

$\begin{align} \nabla\cdot\vec A &=\nabla\cdot(\phi\nabla\psi \;-\; \psi\nabla\phi)\\ &=(\nabla\phi)\cdot(\nabla\psi) \;+\; \phi\nabla^2\psi \;-\; (\nabla\phi)\cdot(\nabla\psi) \;-\; \psi\nabla^2\phi\\ &=\phi\nabla^2\psi \;-\; \psi\nabla^2\phi. \end{align}$

Plugging this into the divergence theorem produces Green's theorem:

Suppose that the linear differential operator L is the Laplacian, and that there is a Green's function G for the Laplacian. The defining property of the Green's function still holds:

Let in Green's theorem. Then:

$\begin{align} & {} \quad \int_V \left\ d^3x' \\ & = \int_S \left \cdot d\hat\sigma'. \end{align}$

Using this expression, it is possible to solve Laplace's equation or Poisson's equation, subject to either Neumann or Dirichlet boundary conditions. In other words, we can solve for everywhere inside a volume where either (1) the value of is specified on the bounding surface of the volume (Dirichlet boundary conditions), or (2) the normal derivative of is specified on the bounding surface (Neumann boundary conditions).

Suppose the problem is to solve for inside the region. Then the integral

reduces to simply due to the defining property of the Dirac delta function and we have:

This form expresses the well-known property of harmonic functions that if the value or normal derivative is known on a bounding surface, then the value of the function inside the volume is known everywhere.

In electrostatics, is interpreted as the electric potential, as electric charge density, and the normal derivative as the normal component of the electric field.

If the problem is to solve a Dirichlet boundary value problem, the Green's function should be chosen such that vanishes when either x or x' is on the bounding surface.Thus only one of the two terms in the surface integral remains. If the problem is to solve a Neumann boundary value problem, the Green's function is chosen such that its normal derivative vanishes on the bounding surface, as it would seems to be the most logical choice. (See Jackson J.D. classical electrodynamics, page 39). However, application of Gauss's theorem to the differential equation defining the Green's function yields

meaning the normal derivative of cannot vanish on the surface, because it must integrate to 1 on the surface. (Again, see Jackson J.D. classical electrodynamics, page 39 for this and the following argument). The simplest form the normal derivative can take is that of a constant, namely, where S is the surface area of the surface. The surface term in the solution becomes

where is the average value of the potential on the surface. This number is not known in general, but is often unimportant, as the goal is often to obtain the electric field given by the gradient of the potential, rather than the potential itself.

With no boundary conditions, the Green's function for the Laplacian (Green's function for the three-variable Laplace equation) is:

Supposing that the bounding surface goes out to infinity, and plugging in this expression for the Green's function, this gives the familiar expression for electric potential in terms of electric charge density as

Read more about this topic: Green's Function

Famous quotes containing the words green and/or functions:

“When every Sunday afternoon
On the Green Lands I walk
And wear a coat in fashion,
Memories of the talk
Of hen wives and of queer old men
Brace me and make me strong....”
—William Butler Yeats (1865–1939)

“Nobody is so constituted as to be able to live everywhere and anywhere; and he who has great duties to perform, which lay claim to all his strength, has, in this respect, a very limited choice. The influence of climate upon the bodily functions ... extends so far, that a blunder in the choice of locality and climate is able not only to alienate a man from his actual duty, but also to withhold it from him altogether, so that he never even comes face to face with it.”
—Friedrich Nietzsche (1844–1900)