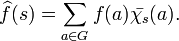Fourier Transform On Finite Groups - Fourier Transform On Finite Abelian Groups

Fourier Transform On Finite Abelian Groups

Since the irreducible representations of finite abelian groups are all of degree 1 and hence equal to the irreducible characters of the group, Fourier analysis on finite abelian groups is significantly simplified. For instance, the Fourier transform yields a scalar- and not matrix-valued function.

Furthermore, the irreducible characters of a group may be put in one-to-one correspondence with the elements of the group.

Therefore, we may define the Fourier transform for finite abelian groups as

$\widehat{f}(s) = \sum_{a \in G} f(a) \bar{\chi_s}(a).$

Note that the right-hand side is simply for the inner product on the vector space of functions from to defined by

$\langle f, g \rangle = \sum_{a \in G} f(a) \bar{g}(a).$

The inverse Fourier transform is then given by

$f(a) = \frac{1}{|G|} \sum_{s \in G} \widehat{f}(s) \chi_s(a).$

A property that is often useful in probability is that the Fourier transform of the uniform distribution is simply where 0 is the group identity and is the Kronecker delta.

Read more about this topic: Fourier Transform On Finite Groups

Famous quotes containing the words transform, finite and/or groups:

“The lullaby is the spell whereby the mother attempts to transform herself back from an ogre to a saint.”
—James Fenton (b. 1949)

“Are not all finite beings better pleased with motions relative than absolute?”
—Henry David Thoreau (1817–1862)

“If we can learn ... to look at the ways in which various groups appropriate and use the mass-produced art of our culture ... we may well begin to understand that although the ideological power of contemporary cultural forms is enormous, indeed sometimes even frightening, that power is not yet all-pervasive, totally vigilant, or complete.”
—Janice A. Radway (b. 1949)