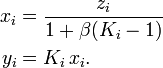Flash Evaporation - Equilibrium Flash of A Multi-component Liquid

Equilibrium Flash of A Multi-component Liquid

The equilibrium flash of a multi-component liquid may be visualized as a simple distillation process using a single equilibrium stage. It is very different and more complex than the flash evaporation of single-component liquid. For a multi-component liquid, calculating the amounts of flashed vapor and residual liquid in equilibrium with each other at a given temperature and pressure requires a trial-and-error iterative solution. Such a calculation is commonly referred to as an equilibrium flash calculation. It involves solving the Rachford-Rice equation:

where:

  • zi is the mole fraction of component i in the feed liquid (assumed to be known);
  • β is the fraction of feed that is vaporised;
  • Ki is the equilibrium constant of component i.

The equilibrium constants Ki are in general functions of many parameters, though the most important is arguably temperature; they are defined as:

where:

  • xi is the mole fraction of component i in liquid phase;
  • yi is the mole fraction of component i in gas phase.

Once the Rachford-Rice equation has been solved for β, the compositions xi and yi can be immediately calculated as:

\begin{align} x_i &= \frac{z_i}{1+\beta(K_i-1)}\\ y_i &= K_i\,x_i. \end{align}

The Rachford-Rice equation can have multiple solutions for β, at most one of which guarantees that all xi and yi will be positive. In particular, if there is only one β for which:

then that β is the solution; if there are multiple such β's, it means that either Kmax<1 or Kmin>1, indicating respectively that no gas phase can be sustained (and therefore β=0) or conversely that no liquid phase can exist (and therefore β=1).

It is possible to use Newton's method for solving the above water equation, but there is a risk of converging to the wrong value of β; it is important to initialise the solver to a sensible initial value, such as (βmax+βmin)/2 (which is however not sufficient: Newton's method makes no guarantees on stability), or, alternatively, use a bracketing solver such as the bisection method or the Brent method, which are guaranteed to converge but can be slower.

The equilibrium flash of multi-component liquids is very widely utilized in petroleum refineries, petrochemical and chemical plants and natural gas processing plants.

Read more about this topic:  Flash Evaporation

Famous quotes containing the words equilibrium, flash and/or liquid:

    “There is a relation between the hours of our life and the centuries of time. As the air I breathe is drawn from the great repositories of nature, as the light on my book is yielded by a star a hundred millions of miles distant, as the poise of my body depends on the equilibrium of centrifugal and centripetal forces, so the hours should be instructed by the ages and the ages explained by the hours.”
    —Ralph Waldo Emerson (1803–1882)

    “They flash upon that inward eye
    Which is the bliss of solitude;
    And then my heart with pleasure fills,
    And dances with the daffodils.”
    —William Wordsworth (1770–1850)

    “Don’t forget the Dance Halls
    Warwick and Savoy,
    Where he picked his women, where
    He drank his liquid joy.”
    —Gwendolyn Brooks (b. 1917)