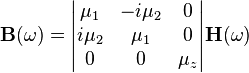Mathematical Formulation
Formally, the magnetic permeability is treated as a non-diagonal tensor as expressed by the equation::
The relation between the angle of rotation of the polarization and the magnetic field in a transparent material is:
where
- β is the angle of rotation (in radians)
- B is the magnetic flux density in the direction of propagation (in teslas)
- d is the length of the path (in meters) where the light and magnetic field interact
- is the Verdet constant for the material. This empirical proportionality constant (in units of radians per tesla per meter) varies with wavelength and temperature and is tabulated for various materials.
A positive Verdet constant corresponds to L-rotation (anticlockwise) when the direction of propagation is parallel to the magnetic field and to R-rotation (clockwise) when the direction of propagation is anti-parallel. Thus, if a ray of light is passed through a material and reflected back through it, the rotation doubles.
Some materials, such as terbium gallium garnet (TGG) have extremely high Verdet constants (≈ −40 rad T−1 m−1). By placing a rod of this material in a strong magnetic field, Faraday rotation angles of over 0.78 rad (45°) can be achieved. This allows the construction of Faraday rotators, which are the principal component of Faraday isolators, devices which transmit light in only one direction.
Similar isolators are constructed for microwave systems by using ferrite rods in a waveguide with a surrounding magnetic field.
Read more about this topic: Faraday Effect
Famous quotes containing the words mathematical and/or formulation:
“The circumstances of human society are too complicated to be submitted to the rigour of mathematical calculation.”
—Marquis De Custine (1790–1857)
“Art is an experience, not the formulation of a problem.”
—Lindsay Anderson (b. 1923)