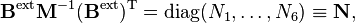Eckart Conditions - Vibrational Energy

Vibrational Energy

The vibrational energy of the molecule can be written in terms of coordinates with respect to the Eckart frame as

$2T_\mathrm{vib} = \sum_{A=1}^N M_A \dot{\mathbf{R}}_A\cdot \dot{\mathbf{R}}_A = \sum_{A=1}^N M_A \dot{\mathbf{d}}_A\cdot \dot{\mathbf{d}}_A.$

Because the Eckart frame is non-inertial, the total kinetic energy comprises also centrifugal and Coriolis energies. These stay out of the present discussion. The vibrational energy is written in terms of the displacement coordinates, which are linearly dependent because they are contaminated by the 6 external modes, which are zero, i.e., the d_A's satisfy 6 linear relations. It is possible to write the vibrational energy solely in terms of the internal modes q_r (r =1, ..., 3N-6) as we will now show. We write the different modes in terms of the displacements

$\begin{align} q_r = \sum_{Aj} d_{Aj}& \big( q^A_{rj} \big) \\ s_i = \sum_{Aj} d_{Aj}& \big( M_A \delta_{ij} \big) =0 \\ s_{i+3} = \sum_{Aj} d_{Aj}& \big( M_A \sum_k \epsilon_{ikj} R^0_{Ak} \big)=0 \\ \end{align}$

The parenthesized expressions define a matrix B relating the internal and external modes to the displacements. The matrix B may be partitioned in an internal (3N-6 x 3N) and an external (6 x 3N) part,

$\mathbf{v}\equiv \begin{pmatrix} q_1 \\ \vdots \\ \vdots \\ q_{3N-6} \\ 0 \\ \vdots \\ 0\\ \end{pmatrix} = \begin{pmatrix} \mathbf{B}^\mathrm{int} \\ \cdots \\ \mathbf{B}^\mathrm{ext} \\ \end{pmatrix} \mathbf{d} \equiv \mathbf{B} \mathbf{d}.$

We define the matrix M by

$\mathbf{M} \equiv \operatorname{diag}(\mathbf{M}_1, \mathbf{M}_2, \ldots,\mathbf{M}_N) \quad\textrm{and}\quad \mathbf{M}_A\equiv \operatorname{diag}(M_A, M_A, M_A)$

and from the relations given in the previous sections follow the matrix relations

$\mathbf{B}^\mathrm{ext} \mathbf{M}^{-1} (\mathbf{B}^\mathrm{ext})^\mathrm{T} = \operatorname{diag}(N_1,\ldots, N_6) \equiv\mathbf{N},$

and

$\mathbf{B}^\mathrm{int} \mathbf{M}^{-1} (\mathbf{B}^\mathrm{ext})^\mathrm{T} = \mathbf{0}.$

We define

$\mathbf{G} \equiv \mathbf{B}^\mathrm{int} \mathbf{M}^{-1} (\mathbf{B}^\mathrm{int})^\mathrm{T}.$

By using the rules for block matrix multiplication we can show that

$(\mathbf{B}^\mathrm{T})^{-1} \mathbf{M} \mathbf{B}^{-1} = \begin{pmatrix} \mathbf{G}^{-1} && \mathbf{0} \\ \mathbf{0} && \mathbf{N}^{-1} \end{pmatrix},$

where G−1 is of dimension (3N-6 x 3N-6) and N−1 is (6 x 6). The kinetic energy becomes

$2T_\mathrm{vib} = \dot{\mathbf{d}}^\mathrm{T} \mathbf{M} \dot{\mathbf{d}} = \dot{\mathbf{v}}^\mathrm{T}\; (\mathbf{B}^\mathrm{T})^{-1} \mathbf{M} \mathbf{B}^{-1}\; \dot{\mathbf{v}} = \sum_{r, r'=1}^{3N-6} (G^{-1})_{r r'} \dot{q}_r \dot{q}_{r'}$

where we used that the last 6 components of v are zero. This form of the kinetic energy of vibration enters Wilson's GF method. It is of some interest to point out that the potential energy in the harmonic approximation can be written as follows

$2V_\mathrm{harm} = \mathbf{d}^\mathrm{T} \mathbf{H} \mathbf{d} = \mathbf{v}^\mathrm{T} (\mathbf{B}^\mathrm{T})^{-1} \mathbf{H} \mathbf{B}^{-1} \mathbf{v} = \sum_{r, r'=1}^{3N-6} F_{r r'} q_r q_{r'},$

where H is the Hessian of the potential in the minimum and F, defined by this equation, is the F matrix of the GF method.

Read more about this topic: Eckart Conditions

Famous quotes containing the word energy:

“Viewed narrowly, all life is universal hunger and an expression of energy associated with it.”
—Mary Ritter Beard (1876–1958)