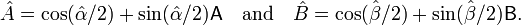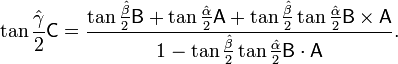Dual Quaternion - Dual Quaternions and Spatial Displacements | Dual Quaternions Spatial Displacements

Dual Quaternions and Spatial Displacements

A benefit of the dual quaternion formulation of the composition of two spatial displacements D_B=(, b) and D_A=(,a) is that the resulting dual quaternion yields directly the screw axis and dual angle of the composite displacement D_C=D_BD_A.

In general, the dual quaternion associated with a spatial displacement D = (,d) is constructed from its screw axis S={S, V) and the dual angle (φ, d) where φ is the rotation about and d the slide along this axis, which defines the displacement D. The associated dual quaternion is given by,

Let the composition of the displacement D_B with D_A be the displacement D_C=D_BD_A. The screw axis and dual angle of D_C is obtained from the product of the dual quaternions of D_A and D_B, given by

$\hat{A}=\cos(\hat{\alpha}/2)+ \sin(\hat{\alpha}/2)\mathsf{A}\quad \text{and}\quad \hat{B}=\cos(\hat{\beta}/2)+ \sin(\hat{\beta}/2)\mathsf{B}.$

That is, the composite displacement D_C=D_BD_A has the associated dual quaternion given by

$\hat{C} = \cos\frac{\hat{\gamma}}{2}+\sin\frac{\hat{\gamma}}{2}\mathsf{C} = \Big(\cos\frac{\hat{\beta}}{2}+\sin\frac{\hat{\beta}}{2}\mathsf{B}\Big) \Big(\cos\frac{\hat{\alpha}}{2}+ \sin\frac{\hat{\alpha}}{2}\mathsf{A}\Big).$

Expand this product in order to obtain

$\cos\frac{\hat{\gamma}}{2}+\sin\frac{\hat{\gamma}}{2} \mathsf{C} = \Big(\cos\frac{\hat{\beta}}{2}\cos\frac{\hat{\alpha}}{2} - \sin\frac{\hat{\beta}}{2}\sin\frac{\hat{\alpha}}{2} \mathsf{B}\cdot \mathsf{A}\Big) + \Big(\sin\frac{\hat{\beta}}{2}\cos\frac{\hat{\alpha}}{2} \mathsf{B} + \sin\frac{\hat{\alpha}}{2}\cos\frac{\hat{\beta}}{2} \mathsf{A} + \sin\frac{\hat{\beta}}{2}\sin\frac{\hat{\alpha}}{2} \mathsf{B}\times \mathsf{A}\Big).$

Divide both sides of this equation by the identity

$\cos\frac{\hat{\gamma}}{2} = \cos\frac{\hat{\beta}}{2}\cos\frac{\hat{\alpha}}{2} - \sin\frac{\hat{\beta}}{2}\sin\frac{\hat{\alpha}}{2} \mathsf{B}\cdot \mathsf{A}$

to obtain

$\tan\frac{\hat{\gamma}}{2} \mathsf{C} = \frac{\tan\frac{\hat{\beta}}{2}\mathsf{B} + \tan\frac{\hat{\alpha}}{2} \mathsf{A} + \tan\frac{\hat{\beta}}{2}\tan\frac{\hat{\alpha}}{2} \mathsf{B}\times \mathsf{A}}{1 - \tan\frac{\hat{\beta}}{2}\tan\frac{\hat{\alpha}}{2} \mathsf{B}\cdot \mathsf{A}}.$

This is Rodrigues formula for the screw axis of a composite displacement defined in terms of the screw axes of the two displacements. He derived this formula in 1840.

The three screw axes A, B, and C form a spatial triangle and the dual angles at these vertices between the common normals that form the sides of this triangle are directly related to the dual angles of the three spatial displacements.

Read more about this topic: Dual Quaternion

Famous quotes containing the word dual:

“Thee for my recitative,
Thee in the driving storm even as now, the snow, the winter-day
declining,
Thee in thy panoply, thy measur’d dual throbbing and thy beat
convulsive,
Thy black cylindric body, golden brass and silvery steel,”
—Walt Whitman (1819–1892)