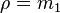Directional Statistics - Moments

Moments

The raw vector (or trigonometric) moments of a circular distribution are defined as

m_n=E(z^n)=\int_\Gamma P(\theta)z^n d\theta\,

where is any interval of length and is the PDF of the circular distribution. Since the integral is unity, and the integration interval is finite, it follows that the moments of any circular distribution are always finite and well defined.

Sample moments are analogously defined:

\overline{m}_n=\frac{1}{N}\sum_{i=1}^N z_i^n.

The population resultant vector, length, and mean angle are defined in analogy with the corresponding sample parameters.

\rho=m_1\,
R=|m_1|\,
\theta_\mu=\mathrm{Arg}(m_1).\,

In addition, the lengths of the higher moments are defined as:

R_n=|m_n|\,

while the angular parts of the higher moments are just . The lengths of the higher moments will all lie between 0 and 1.

Read more about this topic:  Directional Statistics

Famous quotes containing the word moments:

    “The dissenter is every human being at those moments of his life when he resigns momentarily from the herd and thinks for himself.”
    —Archibald MacLeish (1892–1982)

    “I mean, we all have moments of deja vu, but this was ridiculous.”
    —Stanley Kubrick (b. 1928)

    “The woman and the genius do not work. Up to now, woman has been mankind’s supreme luxury. In all those moments when we do our best, we do not work. Work is merely a means to these moments.”
    —Friedrich Nietzsche (1844–1900)