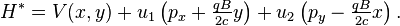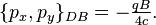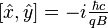Dirac Bracket - Illustration On The Example Provided

Illustration On The Example Provided

Returning to the above example, the naive Hamiltonian and the two primary constraints are

H = V(x, y)
\phi_1 = p_x + \tfrac{q B}{2c} y,\qquad \phi_2 = p_y - \tfrac{q B}{2 c} x.

Therefore the extended Hamiltonian can be written

H^* = V(x, y) + u_1 \left(p_x + \tfrac{q B}{2c}y\right) + u_2 \left(p_y - \tfrac{q B}{2c}x\right).

The next step is to apply the consistency conditions, which in this case become

\{\phi_1, H\}_{PB}+\sum_j u_j\{\phi_1, \phi_j\}_{PB} = -\frac{\partial V}{\partial x} + u_2 \frac{q B}{c} \approx 0
\{\phi_2, H\}_{PB}+\sum_j u_j\{\phi_2, \phi_j\}_{PB} = -\frac{\partial V}{\partial y} - u_1 \frac{q B}{c} \approx 0.

These are not secondary constraints, but conditions that fix and . Therefore, there are no secondary constraints and the arbitrary coefficients are completely determined, indicating that there are no unphysical degrees of freedom.

If one plugs in with the values of and, then one can see that the equations of motion are

\dot{x} = \{x, H\}_{PB} + u_1\{x, \phi_1\}_{PB} + u_2 \{x, \phi_2\} = -\frac{c}{q B} \frac{\partial V}{\partial y}
\dot{y} = \frac{c}{q B} \frac{\partial V}{\partial x}
\dot{p}_x = -\frac{1}{2}\frac{\partial V}{\partial x}
\dot{p}_y = -\frac{1}{2}\frac{\partial V}{\partial y},

which are self-consistent and coincide with the Lagrangian equations of motion.

A simple calculation confirms that and are second class constraints since

\{\phi_1, \phi_2\}_{PB} = - \{\phi_2, \phi_1\}_{PB} = \frac{q B}{c},

hence the matrix looks like

M = \frac{q B}{c} \left(\begin{matrix} 0 & 1\\ -1 & 0 \end{matrix}\right),

which is easily inverted to

M^{-1} = \frac{c}{q B} \left(\begin{matrix} 0 & -1\\ 1 & 0 \end{matrix}\right) \quad\Rightarrow\quad M^{-1}_{ab} = -\frac{c}{q B_0} \epsilon_{ab},

where is the Levi-Civita symbol. Thus, the Dirac brackets are defined to be

\{f, g\}_{DB} = \{f, g\}_{PB} + \frac{c\epsilon_{ab}}{q B} \{f, \phi_a\}_{PB}\{\phi_b, g\}_{PB}.

If one always uses the Dirac bracket instead of the Poisson bracket then there is no issue about the order of applying constraints and evaluating expressions, since the Dirac bracket of anything weakly zero is strongly equal to zero. This means that one can just use the naive Hamiltonian with Dirac brackets, and get the correct equations of motion, which one can easily confirm.

To quantize the system, the Dirac brackets between all of the phase space variables are needed. The nonvanishing Dirac brackets for this system are

\{x, y\}_{DB} = -\tfrac{c}{q B}
\{x, p_x\}_{DB} = \{y, p_y\}_{DB} = \frac{1}{2}

while the cross-terms vanish, and

\{p_x, p_y\}_{DB} = - \tfrac{q B}{4c}.

Therefore, the correct implementation of canonical quantization dictates the commutation relations,

= -i\tfrac{\hbar c}{q B}
= = i\frac{\hbar}{2}

with the cross terms vanishing, and

= -i\tfrac{\hbar q B}{4c}~.

Interestingly, this example has a nonvanishing commutator between and, which means this structure specifies a noncommutative geometry. (Since the two coordinates do not commute, there will be an uncertainty principle for the x and y positions.)

Similarly, for free motion on a hypersphere Sn, the n+1 coordinates are constrained . From a plain kinetic Lagrangian, it is evident that their momenta are perpendicular to them, . Thus the corresponding Dirac Brackets are likewise simple to work out,

\{x_i, x_j\}_{DB} = 0,
\{x_i, p_j\}_{DB} = \delta_{ij} -x_i x_j ,
\{p_i, p_j\}_{DB} = x_j p_i - x_i p_j ~.

The 2(n+1) constrained phase-space variables (xi, pi) obey much simpler Dirac brackets than the 2n unconstrained variables, had one eliminated one of the xs and one of the ps through the two constraints ab initio, would obey plain Poisson brackets. The Dirac brackets add simplicity and elegance, at the cost of excessive (constrained) phase-space variables.

Read more about this topic:  Dirac Bracket

Famous quotes containing the word illustration:

    “What is character but the determination of incident? What is incident but the illustration of character?”
    —Henry James (1843–1916)