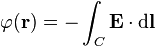Classical Electromagnetism - The Electric Field E

The Electric Field E

The electric field E is defined such that, on a stationary charge:

\mathbf{F} = q_0 \mathbf{E}

where q0 is what is known as a test charge. The size of the charge doesn't really matter, as long as it is small enough not to influence the electric field by its mere presence. What is plain from this definition, though, is that the unit of E is N/C (newtons per coulomb). This unit is equal to V/m (volts per meter), see below.

In electrostatics, where charges are not moving, around a distribution of point charges, the forces determined from Coulomb's law may be summed. The result after dividing by q0 is:

where n is the number of charges, qi is the amount of charge associated with the ith charge, ri is the position of the ith charge, r is the position where the electric field is being determined, and ε0 is the electric constant.

If the field is instead produced by a continuous distribution of charge, the summation becomes an integral:

where is the charge density and is the vector that points from the volume element to the point in space where E is being determined.

Both of the above equations are cumbersome, especially if one wants to determine E as a function of position. A scalar function called the electric potential can help. Electric potential, also called voltage (the units for which are the volt), is defined by the line integral

\varphi \mathbf{(r)} = - \int_C \mathbf{E} \cdot \mathrm{d}\mathbf{l}

where φ(r) is the electric potential, and C is the path over which the integral is being taken.

Unfortunately, this definition has a caveat. From Maxwell's equations, it is clear that ∇ × E is not always zero, and hence the scalar potential alone is insufficient to define the electric field exactly. As a result, one must add a correction factor, which is generally done by subtracting the time derivative of the A vector potential described below. Whenever the charges are quasistatic, however, this condition will be essentially met.

From the definition of charge, one can easily show that the electric potential of a point charge as a function of position is:

\varphi \mathbf{(r)} = \frac{1}{4 \pi \varepsilon_0 } \sum_{i=1}^{n} \frac{q_i \left( \mathbf{r} - \mathbf{r}_i \right)} {\left| \mathbf{r} - \mathbf{r}_i \right|}

where q is the point charge's charge, r is the position at which the potential is being determined, and ri is the position of each point charge. The potential for a continuous distribution of charge is:

\varphi \mathbf{(r)} = \frac{1}{4 \pi \varepsilon_0} \int \frac{\rho(\mathbf{r'})}{|\mathbf{r}-\mathbf{r'}|}\, \mathrm{d^3}\mathbf{r'}

where is the charge density, and is the distance from the volume element to point in space where φ is being determined.

The scalar φ will add to other potentials as a scalar. This makes it relatively easy to break complex problems down in to simple parts and add their potentials. Taking the definition of φ backwards, we see that the electric field is just the negative gradient (the del operator) of the potential. Or:

From this formula it is clear that E can be expressed in V/m (volts per meter).

Read more about this topic:  Classical Electromagnetism

Famous quotes containing the words electric and/or field:

    “A sociosphere of contact, control, persuasion and dissuasion, of exhibitions of inhibitions in massive or homeopathic doses...: this is obscenity. All structures turned inside out and exhibited, all operations rendered visible. In America this goes all the way from the bewildering network of aerial telephone and electric wires ... to the concrete multiplication of all the bodily functions in the home, the litany of ingredients on the tiniest can of food, the exhibition of income or IQ.”
    —Jean Baudrillard (b. 1929)

    “In the field of world policy I would dedicate this Nation to the policy of the Good Neighbor—the neighbor who resolutely respects himself and, because he does, respects the rights of others—the neighbor who respects his obligations and respects the sanctity of his agreements in and with a world of neighbors.”
    —Franklin D. Roosevelt (1882–1945)