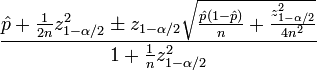Binomial Proportion Confidence Interval - Wilson Score Interval

Wilson Score Interval

The Wilson interval is an improvement (the actual coverage probability is closer to the nominal value) over the normal approximation interval and was first developed by Edwin Bidwell Wilson (1927).

\frac{{ {\hat p + \frac{{1}}{{2n}} z_{1- \alpha / 2}^2 \pm z_{1- \alpha / 2} \sqrt {\frac{{\hat p\left( {1 - \hat p} \right)}}{n} + \frac{{z_{1- \alpha / 2}^2}} {{4n^2}} }} }} {{ {1 + \frac{{1}}{n}} z_{1- \alpha / 2}^2 }}

This interval has good properties even for a small number of trials and/or an extreme probability. The center of the Wilson interval

\frac{{ {\hat p + \frac{{1}}{{2n}} z_{1- \alpha / 2}^2 } }} {{ {1 + \frac{{1}}{n}} z_{1- \alpha / 2}^2 }}

can be shown to be a weighted average of and, with receiving greater weight as the sample size increases. For the 95% interval, the Wilson interval is nearly identical to the normal approximation interval using instead of .

The Wilson interval can be derived from Pearson's chi-squared test with two categories. The resulting interval

can then be solved for to produce the Wilson interval.

The test in the middle of the inequality is a score test, so the Wilson interval is sometimes called the Wilson score interval.

Read more about this topic:  Binomial Proportion Confidence Interval

Famous quotes containing the words wilson, score and/or interval:

    “If you would be a leader of men you must lead your own generation, not the next. Your playing must be good now, while the play is on the boards and the audience in the seats.... It will not get you the repute of a good actor to have excellencies discovered in you afterwards.”
    —Woodrow Wilson (1856–1924)

    “Whereas, before, our forefathers had no other books but the score and the tally, thou hast caused printing to be used, and, contrary to the King, his crown, and dignity, thou hast built a paper-mill.”
    —William Shakespeare (1564–1616)

    “The yearning for an afterlife is the opposite of selfish: it is love and praise for the world that we are privileged, in this complex interval of light, to witness and experience.”
    —John Updike (b. 1932)