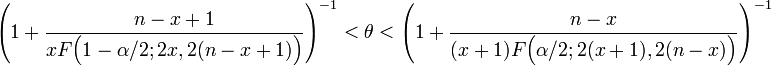Binomial Proportion Confidence Interval - Clopper-Pearson Interval

Clopper-Pearson Interval

The Clopper-Pearson interval is an early and very common method for calculating binomial confidence intervals. This is often called an 'exact' method, but that is because it is based on the cumulative probabilities of the binomial distribution (i.e. exactly the correct distribution rather than an approximation), but the intervals are not exact in the way that one might assume: the discontinuous nature of the binomial distribution precludes any interval with exact coverage for all population proportions. The Clopper-Pearson interval can be written as

where X is the number of successes observed in the sample and Bin(n; θ) is a binomial random variable with n trials and probability of success θ.

Because of a relationship between the cumulative binomial distribution and the beta distribution, the Clopper-Pearson interval is sometimes presented in an alternate format that uses quantiles from the beta distribution.

where x is the number of successes, n is the number of trials, and B(p; v,w) is the pth quantile from a beta distribution with shape parameters v and w. The beta distribution is, in turn, related to the F-distribution so a third formulation of the Clopper-Pearson interval can be written using F percentiles:

where x is the number of successes, n is the number of trials, and F(c; d1, d2) is the 1 - c quantile from an F-distribution with d1 and d2 degrees of freedom.

The Clopper-Pearson interval is an exact interval since it is based directly on the binomial distribution rather than any approximation to the binomial distribution. This interval never has less than the nominal coverage for any population proportion, but that means that it is usually conservative. For example, the true coverage rate of a 95% Clopper-Pearson interval may be well above 95%, depending on n and θ. Thus the interval may be wider than it needs to be to achieve 95% confidence. In contrast, it is worth noting that other confidence bounds may be narrower than their nominal confidence with, i.e., the Normal Approximation (or "Standard") Interval, Wilson Interval, Agresti-Coull Interval, etc., with a nominal coverage of 95% may in fact cover less than 95%.