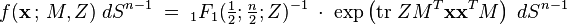Bingham Distribution

In statistics, the Bingham distribution, named after Christopher Bingham, is an antipodally symmetric probability distribution on the n-sphere. It is widely used in paleomagnetic data analysis.

Its probability density function is given by

f(\mathbf{x}\,;\,M,Z)\; dS^{n-1} \;=\; {}_{1}F_{1}({\textstyle\frac{1}{2}};{\textstyle\frac{n}{2}};Z)^{-1}\;\cdot\; \exp\left({\textrm{tr}\; Z M^{T}\mathbf{x} \mathbf{x}^{T}M}\right)\; dS^{n-1}

which may also be written

f(\mathbf{x}\,;\,M,Z)\; dS^{n-1} \;=\; {}_{1}F_{1}({\textstyle\frac{1}{2}};{\textstyle\frac{n}{2}};Z)^{-1}\;\cdot\; \exp\left({\mathbf{x}^{T} M Z M^{T}\mathbf{x} }\right)\; dS^{n-1}

where x is an axis, M is an orthogonal orientation matrix, Z is a diagonal concentration matrix, is a confluent hypergeometric function of matrix argument.

Read more about Bingham Distribution:  See Also

Famous quotes containing the words bingham and/or distribution:

    “An isolated outbreak of virginity ... is a rash on the face of society. It arouses only pity from the married, and embarrassment from the single.”
    —Charlotte Bingham (b. 1942)

    “Classical and romantic: private language of a family quarrel, a dead dispute over the distribution of emphasis between man and nature.”
    —Cyril Connolly (1903–1974)