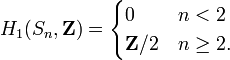Symmetric Group - Homology

Homology

See also: Alternating group#Group homology

The group homology of is quite regular and stabilizes: the first homology (concretely, the abelianization) is:

The first homology group is the abelianization, and corresponds to the sign map S_n → S₂ which is the abelianization for n ≥ 2; for n < 2 the symmetric group is trivial. This homology is easily computed as follows: S_n is generated by involutions (2-cycles, which have order 2), so the only non-trivial maps are to S₂ and all involutions are conjugate, hence map to the same element in the abelianization (since conjugation is trivial in abelian groups). Thus the only possible maps S_n → S₂ ≅ {±1} send an involution to 1 (the trivial map) or to −1 (the sign map). One must also show that the sign map is well-defined, but assuming that, this gives the first homology of S_n.

The second homology (concretely, the Schur multiplier) is:

This was computed in (Schur 1911), and corresponds to the double cover of the symmetric group, 2 · S_n.

Note that the exceptional low-dimensional homology of the alternating group ( corresponding to non-trivial abelianization, and due to the exceptional 3-fold cover) does not change the homology of the symmetric group; the alternating group phenomena do yield symmetric group phenomena – the map extends to and the triple covers of A₆ and A₇ extend to triple covers of S₆ and S₇ – but these are not homological – the map does not change the abelianization of S₄, and the triple covers do not correspond to homology either.

The homology "stabilizes" in the sense of stable homotopy theory: there is an inclusion map and for fixed k, the induced map on homology is an isomorphism for sufficiently high n. This is analogous to the homology of families Lie groups stabilizing.

The homology of the infinite symmetric group is computed in (Nakaoka 1961), with the cohomology algebra forming a Hopf algebra.