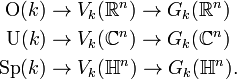Stiefel Manifold - As A Principal Bundle

As A Principal Bundle

There is a natural projection

from the Stiefel manifold Vk(Fn) to the Grassmannian of k-planes in Fn which sends a k-frame to the subspace spanned by that frame. The fiber over a given point P in Gk(Fn) is the set of all orthonormal k-frames contained in the space P.

This projection has the structure of a principal G-bundle where G is the associated classical group of degree k. Take the real case for concreteness. There is a natural right action of O(k) on Vk(Rn) which rotates a k-frame in the space it spans. This action is free but not transitive. The orbits of this action are precisely the orthonormal k-frames spanning a given k-dimensional subspace; that is, they are the fibers of the map p. Similar arguments hold in the complex and quaternionic cases.

We then have a sequence of principal bundles:

\begin{align} \mathrm O(k) &\to V_k(\mathbb R^n) \to G_k(\mathbb R^n)\\ \mathrm U(k) &\to V_k(\mathbb C^n) \to G_k(\mathbb C^n)\\ \mathrm{Sp}(k) &\to V_k(\mathbb H^n) \to G_k(\mathbb H^n). \end{align}

The vector bundles associated to these principal bundles via the natural action of G on Fk are just the tautological bundles over the Grassmannians. In other words, the Stiefel manifold Vk(Fn) is the orthogonal, unitary, or symplectic frame bundle associated to the tautological bundle on a Grassmannian.

When one passes to the n → ∞ limit, these bundles become the universal bundles for the classical groups.

Read more about this topic:  Stiefel Manifold

Famous quotes containing the words principal and/or bundle:

    “... [a] girl one day flared out and told the principal “the only mission opening before a girl in his school was to marry one of those candidates [for the ministry].” He said he didn’t know but it was. And when at last that same girl announced her desire and intention to go to college it was received with about the same incredulity and dismay as if a brass button on one of those candidate’s coats had propounded a new method for squaring the circle or trisecting the arc.”
    —Anna Julia Cooper (1859–1964)

    “We styled ourselves the Knights of the Umbrella and the Bundle; for, wherever we went ... the umbrella and the bundle went with us; for we wished to be ready to digress at any moment. We made it our home nowhere in particular, but everywhere where our umbrella and bundle were.”
    —Henry David Thoreau (1817–1862)