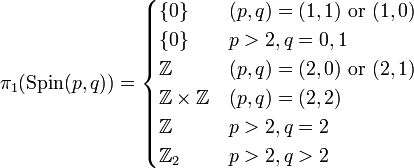Spin Group - Topological Considerations

Topological Considerations

Connected and simply connected Lie groups are classified by their Lie algebra. So if G is a connected Lie group with a simple Lie algebra, with G′ the universal cover of G, there is an inclusion

with Z(G′) the center of G′. This inclusion and the Lie algebra of G determine G entirely (note that it is not the fact that and determine G entirely; for instance SL(2, R) and PSL(2, R) have the same Lie algebra and same fundamental group, but are not isomorphic).

The definite signature Spin(n) are all simply connected for n > 2 , so they are the universal coverings for SO(n).

In indefinite signature, Spin(p, q) is not connected, and in general the identity component, Spin₀(p, q), is not simply connected, thus it is not a universal cover. The fundamental group is most easily understood by considering the maximal compact subgroup of SO(p, q) , which is SO(p) × SO(q), and noting that rather than being the product of the 2-fold covers (hence a 4-fold cover), Spin(p, q) is the "diagonal" 2-fold cover – it is a 2-fold quotient of the 4-fold cover. Explicitly, the maximal compact connected subgroup of Spin(p, q) is

Spin(p) × Spin(q) / {(1, 1), (−1, −1)} .

This allows us to calculate the fundamental groups of Spin(p, q), taking :

$\pi_1(\mbox{Spin}(p,q)) = \begin{cases} \{0\} & (p,q)=(1,1) \mbox{ or } (1,0) \\ \{0\} & p > 2, q = 0,1 \\ \mathbb{Z} & (p,q)=(2,0) \mbox{ or } (2,1) \\ \mathbb{Z} \times \mathbb{Z} & (p,q) = (2,2) \\ \mathbb{Z} & p > 2, q=2 \\ \mathbb{Z}_2 & p >2, q >2 \\ \end{cases}$

Thus once the fundamental group is as it is a 2-fold quotient of a product of two universal covers.

The maps on fundamental groups are given as follows. For , this implies that the map is given by going to . For p = 2 , q > 2 , this map is given by . And finally, for p = q = 2 , is sent to and (0, 1) is sent to (1, −1).