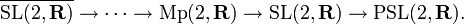SL2(R) - Topology and Universal Cover

Topology and Universal Cover

As a topological space, PSL(2,R) can be described as the unit tangent bundle of the hyperbolic plane. It is a circle bundle, and has a natural contact structure induced by the symplectic structure on the hyperbolic plane. SL(2,R) is a 2-fold cover of PSL(2,R), and can be thought of as the bundle of spinors on the hyperbolic plane.

The fundamental group of SL(2,R) is the infinite cyclic group Z. The universal covering group, denoted, is an example of a finite-dimensional Lie group that is not a matrix group. That is, admits no faithful, finite-dimensional representation.

As a topological space, is a line bundle over the hyperbolic plane. When imbued with a left-invariant metric, the 3-manifold becomes one of the eight Thurston geometries. For example, is the universal cover of the unit tangent bundle to any hyperbolic surface. Any manifold modeled on is orientable, and is a circle bundle over some 2-dimensional hyperbolic orbifold (a Seifert fiber space).

Under this covering, the preimage of the modular group PSL(2,Z) is the braid group on 3 generators, B₃, which is the universal central extension of the modular group. These are lattices inside the relevant algebraic groups, and this corresponds algebraically to the universal covering group in topology.

The 2-fold covering group can be identified as Mp(2,R), a metaplectic group, thinking of SL(2,R) as the symplectic group Sp(2,R).

The aforementioned groups together form a sequence:

However, there are other covering groups of PSL(2,R) corresponding to all n, as n Z < Z ≅ π₁ (PSL(2,R)), which form a lattice of covering groups by divisibility; these cover SL(2,R) if and only if n is even.