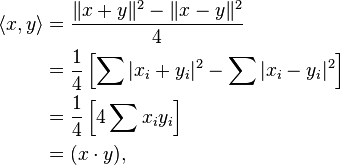Parallelogram Law - Normed Vector Spaces Satisfying The Parallelogram Law

Normed Vector Spaces Satisfying The Parallelogram Law

Most real and complex normed vector spaces do not have inner products, but all normed vector spaces have norms (by definition). For example, a commonly used norm is the p-norm:

where the are the components of vector .

Given a norm, one can evaluate both sides of the parallelogram law above. A remarkable fact is that if the parallelogram law holds, then the norm must arise in the usual way from some inner product. In particular, it holds for the p-norm if and only if p = 2, the so-called Euclidean norm or standard norm.

For any norm satisfying the parallelogram law (which necessarily is an inner product norm), the inner product generating the norm is unique as a consequence of the polarization identity. In the real case, the polarization identity is given by:

or, equivalently, by:

In the complex case it is given by:

For example, using the p-norm with p = 2 and real vectors, the evaluation of the inner product proceeds as follows:

\begin{align} \langle x, y\rangle&={\|x+y\|^2-\|x-y\|^2\over 4}\\ &=\frac{1}{4} \left\\ &=\frac{1}{4} \left\\ &=(x\cdot y), \end{align}

which is the standard dot product of two vectors.

Read more about this topic:  Parallelogram Law

Famous quotes containing the words spaces, satisfying and/or law:

    “Le silence éternel de ces espaces infinis m’effraie. The eternal silence of these infinite spaces frightens me.”
    —Blaise Pascal (1623–1662)

    “He was always a seeker after something in the world that is there in no satisfying measure, or not at all.”
    —Walter Pater (1839–1894)

    “I was not born to be forced. I will breathe after my own fashion. Let us see who is the strongest. What force has a multitude? They can only force me who obey a higher law than I.... I do not hear of men being forced to live this way or that by masses of men. What sort of life were that to live?”
    —Henry David Thoreau (1817–1862)