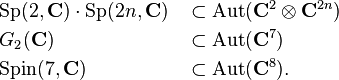Holonomy - Affine Holonomy

Affine Holonomy

Affine holonomy groups are the groups arising as holonomies of torsion-free affine connections; those which are not Riemannian or pseudo-Riemannian holonomy groups are also known as non-metric holonomy groups. The deRham decomposition theorem does not apply to affine holonomy groups, so a complete classification is out of reach. However, it is still natural to classify irreducible affine holonomies.

On the way to his classification of Riemannian holonomy groups, Berger developed two criteria that must be satisfied by the Lie algebra of the holonomy group of a torsion-free affine connection which is not locally symmetric: one of them, known as Berger's first criterion, is a consequence of the Ambrose–Singer theorem, that the curvature generates the holonomy algebra; the other, known as Berger's second criterion, comes from the requirement that the connection should not be locally symmetric. Berger presented a list of groups acting irreducibly and satisfying these two criteria; this can be interpreted as a list of possibilities for irreducible affine holonomies.

Berger's list was later shown to be incomplete: further examples were found by R. Bryant (1991) and by Q. Chi, S. Merkulov, and L. Schwachhöfer (1996). These are sometimes known as exotic holonomies. The search for examples ultimately led to a complete classification of irreducible affine holonomies by Merkulov and Schwachhöfer (1999), with Bryant (2000) showing that every group on their list occurs as an affine holonomy group.

The Merkulov–Schwachhöfer classification has been clarified considerably by a connection between the groups on the list and certain symmetric spaces, namely the hermitian symmetric spaces and the quaternion-Kähler symmetric spaces. The relationship is particularly clear in the case of complex affine holonomies, as demonstrated by Schwachhöfer (2001).

Let V be a finite dimensional complex vector space, let H ⊂ Aut(V) be an irreducible semisimple complex connected Lie subgroup and let K ⊂ H be a maximal compact subgroup.

1. If there is an irreducible hermitian symmetric space of the form G/(U(1) · K), then both H and C*· H are non-symmetric irreducible affine holonomy groups, where V the tangent representation of K.
2. If there is an irreducible quaternion-Kähler symmetric space of the form G/(Sp(1) · K), then H is a non-symmetric irreducible affine holonomy groups, as is C* · H if dim V = 4. Here the complexified tangent representation of Sp(1) · K is C2 ⊗ V, and H preserves a complex symplectic form on V.

These two families yield all non-symmetric irreducible complex affine holonomy groups apart from the following:

Using the classification of hermitian symmetric spaces, the first family gives the following complex affine holonomy groups:

where Z_C is either trivial, or the group C*.

Using the classification of quaternion-Kähler symmetric spaces, the second family gives the following complex symplectic holonomy groups:

(In the second row, Z_C must be trivial unless n = 2.)

From these lists, an analogue of Simon's result that Riemannian holonomy groups act transitively on spheres may be observed: the complex holonomy representations are all prehomogeneous vector spaces. A conceptual proof of this fact is not known.

The classification of irreducible real affine holonomies can be obtained from a careful analysis, using the lists above and the fact that real affine holonomies complexify to complex ones.