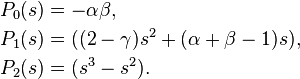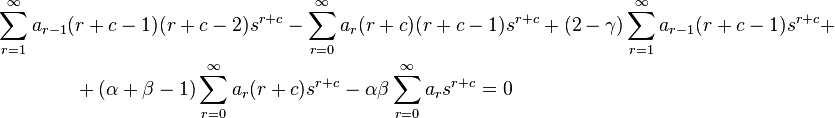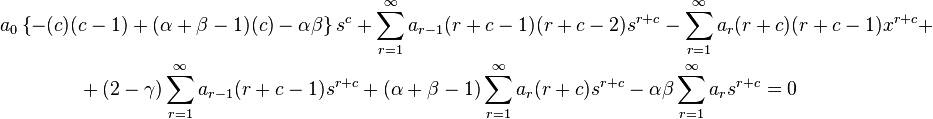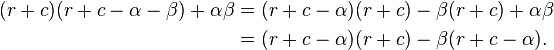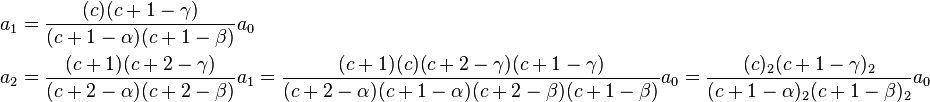Frobenius Solution To The Hypergeometric Equation - Solution Around Infinity

Solution Around Infinity

Finally, we study the singularity as x → ∞. Since we can't study this directly, we let x = s−1. Then the solution of the equation as x → ∞ is identical to the solution of the modified equation when s = 0. We had

\begin{align} & x(1-x)y''+\left\{ \gamma -(1+\alpha +\beta )x \right\}y'-\alpha \beta y=0 \\ & \frac{dy}{dx}=\frac{dy}{ds}\times \frac{ds}{dx}=-s^2\times \frac{dy}{ds}=-s^2y' \\ & \frac{d^{2}y}{dx^{2}}=\frac{d}{dx}\left( \frac{dy}{dx} \right)=\frac{d}{dx}\left( -s^2 \times \frac{dy}{ds} \right)=\frac{d}{ds}\left( -s^2 \times \frac{dy}{ds} \right)\times \frac{ds}{dx} = \left( (-2s)\times \frac{dy}{ds}+(-s^{2})\frac{d^{2}y}{ds^{2}} \right) \times (-s^{2})=2s^{3}y'+s^{4}y'' \end{align}

Hence, the equation takes the new form

which reduces to

Let

\begin{align} P_{0}(s) &=-\alpha \beta, \\ P_{1}(s) &=((2-\gamma )s^{2}+(\alpha +\beta -1)s), \\ P_{2}(s) &=(s^{3}-s^{2}). \end{align}

As we said, we shall only study the solution when s = 0. As we can see, this is a singular point since P2(0) = 0. To see if it's regular,

\begin{align} \lim_{s \to a} \frac{\left( s-a \right)P_{1}(s)}{P_{2}(s)} & =\lim_{s \to 0} \frac{\left( s-0 \right)((2-\gamma )s^{2}+(\alpha +\beta -1)s)}{(s^{3}-s^{2})} \\ &= \lim_{s \to 0} \frac{((2-\gamma )s^{2}+(\alpha +\beta -1)s)}{s^{2}-s} \\ & =\lim_{s \to 0} \frac{((2-\gamma )s+(\alpha +\beta -1))}{s-1}=1-\alpha -\beta. \\ \lim_{s \to a} \frac{\left( s-a \right)^{2}P_{0}(s)}{P_{2}(s)} &=\lim_{s \to 0} \frac{\left( s-0 \right)^{2}\left( -\alpha \beta \right)}{(s^{3}-s^{2})}=\lim_{s \to 0} \frac{\left( -\alpha \beta \right)}{s-1}=\alpha \beta. \end{align}

Hence, both limits exist and s = 0 is a regular singular point. Therefore, we assume the solution takes the form

with a0 ≠ 0.

Hence,

\begin{align} y'&=\sum\limits_{r=0}^{\infty }{a_{r}(r+c)s^{r+c-1}}\\ y''&=\sum\limits_{r=0}^{\infty }{a_{r}(r+c)(r+c-1)s^{r+c-2}} \end{align}

Substituting in the modified hypergeometric equation we get

And therefore:

i.e.,

In order to simplify this equation, we need all powers to be the same, equal to r + c, the smallest power. Hence, we switch the indices as follows

\begin{align} & \sum_{r=1}^{\infty }{a_{r-1}(r+c-1)(r+c-2)s^{r+c}}-\sum_{r=0}^{\infty }{a_{r}(r+c)(r+c-1)s^{r+c}} +(2-\gamma )\sum_{r=1}^{\infty }{a_{r-1}(r+c-1)s^{r+c}}+ \\ & \qquad \qquad + (\alpha +\beta -1)\sum_{r=0}^{\infty }{a_{r}(r+c)s^{r+c}}-\alpha \beta \sum_{r=0}^{\infty }{a_{r}s^{r+c}}=0 \end{align}

Thus, isolating the first term of the sums starting from 0 we get

\begin{align} & a_{0}\left\{ -(c)(c-1)+(\alpha +\beta -1)(c)-\alpha \beta \right\}s^{c}+\sum_{r=1}^{\infty }{a_{r-1}(r+c-1)(r+c-2)s^{r+c}} -\sum_{r=1}^{\infty }{a_{r}(r+c)(r+c-1)x^{r+c}}+\\ & \qquad \qquad + (2-\gamma )\sum_{r=1}^{\infty }{a_{r-1}(r+c-1)s^{r+c}} +(\alpha +\beta -1)\sum_{r=1}^{\infty }{a_{r}(r+c)s^{r+c}}-\alpha \beta \sum_{r=1}^{\infty }{a_{r}s^{r+c}}=0 \end{align}

Now, from the linear independence of all powers of s (i.e., of the functions 1, s, s2, ...), the coefficients of sk vanish for all k. Hence, from the first term we have

which is the indicial equation. Since a0 ≠ 0, we have

Hence, c1 = α and c2 = β.

Also, from the rest of the terms we have

Hence,

But

\begin{align} (r+c)(r+c-\alpha -\beta )+\alpha \beta &=(r+c-\alpha )(r+c)-\beta (r+c)+\alpha \beta \\ & =(r+c-\alpha )(r+c)-\beta (r+c-\alpha ). \end{align}

Hence, we get the recurrence relation

Let's now simplify this relation by giving ar in terms of a0 instead of ar−1. From the recurrence relation,

\begin{align} a_1 &=\frac{(c)(c+1-\gamma )}{(c+1-\alpha )(c+1-\beta )}a_{0} \\ a_2 &=\frac{(c+1)(c+2-\gamma )}{(c+2-\alpha )(c+2-\beta )}a_{1}=\frac{(c+1)(c)(c+2-\gamma )(c+1-\gamma )}{(c+2-\alpha )(c+1-\alpha )(c+2-\beta )(c+1-\beta )}a_{0} = \frac{(c)_{2}(c+1-\gamma )_{2}}{(c+1-\alpha )_{2}(c+1-\beta )_{2}}a_{0} \end{align}

As we can see,

Hence, our assumed solution takes the form

We are now ready to study the solutions corresponding to the different cases for c1 − c2 = α − β.

Read more about this topic:  Frobenius Solution To The Hypergeometric Equation

Famous quotes containing the words solution and/or infinity:

    “The Settlement ... is an experimental effort to aid in the solution of the social and industrial problems which are engendered by the modern conditions of life in a great city. It insists that these problems are not confined to any one portion of the city. It is an attempt to relieve, at the same time, the overaccumulation at one end of society and the destitution at the other ...”
    —Jane Addams (1860–1935)

    “As we begin to comprehend that the earth itself is a kind of manned spaceship hurtling through the infinity of space—it will seem increasingly absurd that we have not better organized the life of the human family.”
    —Hubert H. Humphrey (1911–1978)