Total-energy Distribution
The energy distribution of the emitted electrons is important both for scientific experiments that use the emitted electron energy distribution to probe aspects of the emitter surface physics and for the field emission sources used in electron beam instruments such as electron microscopes. In the latter case, the "width" (in energy) of the distribution influences how finely the beam can be focused.
The theoretical explanation here follows the approach of Forbes. If ε denotes the total electron energy relative to the emitter Fermi level, and Kp denotes the kinetic energy of the electron parallel to the emitter surface, then the electron's normal energy εn (sometimes called its "forwards energy") is defined by
- .
Two types of theoretical energy distribution are recognized: the normal-energy distribution (NED), which shows how the energy εn is distributed immediately after emission (i.e., immediately outside the tunneling barrier); and the total-energy distribution, which shows how the total energy ε is distributed. When the emitter Fermi level is used as the reference zero level, both ε and εn can be either positive or negative.
Energy analysis experiments have been made on field emitters since the 1930s. However, only in the late 1950s was it realized (by Young and Mueller) that these experiments always measured the total energy distribution, which is now usually denoted by j(ε). This is also true (or nearly true) when the emission comes from a small field enhancing protrusion on an otherwise flat surface.
To see how the total energy distribution can be calculated within the framework of a Sommerfeld free-electron-type model, look at the P-T energy-space diagram (P-T="parallel-total").
-
Fig. 1. P-T energy-space diagram, showing the region in P-T energy space where traveling-wave electron states exist.
This shows the "parallel kinetic energy" Kp on the horizontal axis and the total energy ε on the vertical axis. An electron inside the bulk metal usually has values of Kp and ε that lie within the lightly shaded area. It can be shown that each element dεdKp of this energy space makes a contribution to the electron current density incident on the inside of the emitter boundary. Here, zS is the universal constant (called here the Sommerfeld supply density):
and is the Fermi-Dirac distribution function:
where T is thermodynamic temperature and kB is Boltzmann's constant.
This element of incident current density sees a barrier of height h given by:
The corresponding escape probability is D(h,F): this may be expanded (approximately) in the form
where DF is the escape probability for a barrier of unreduced height equal to the local work-function φ. Hence, the element dεdKp makes a contribution to the emission current density, and the total contribution made by incident electrons with energies in the elementary range dε is thus
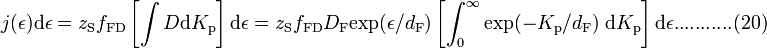 ,
,
where the integral is in principle taken along the strip shown in the diagram, but can in practice be extended to ∞ when the decay-width dF is very much less than the Fermi energy KF (which is always the case for a metal). The outcome of the integration can be written:
where and are values appropriate to a barrier of unreduced height h equal to the local work function φ, and is defined by this equation.
For a given emitter, with a given field applied to it, is independent of F, so eq. (21) shows that the shape of the distribution (as ε increases from a negative value well below the Fermi level) is a rising exponential, multiplied by the FD distribution function. This generates the familiar distribution shape first predicted by Young. At low temperatures, goes sharply from 1 to 0 in the vicinity of the Fermi level, and the FWHM of the distribution is given by:
The fact that experimental CFE total energy distributions have this basic shape is a good experimental confirmation that electrons in metals obey Fermi-Dirac statistics.
Read more about this topic: Field Electron Emission
Famous quotes containing the word distribution:
“My topic for Army reunions ... this summer: How to prepare for war in time of peace. Not by fortifications, by navies, or by standing armies. But by policies which will add to the happiness and the comfort of all our people and which will tend to the distribution of intelligence [and] wealth equally among all. Our strength is a contented and intelligent community.”
—Rutherford Birchard Hayes (1822–1893)