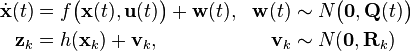Extended Kalman Filter - Discrete-time Extended Kalman Filter

Discrete-time Extended Kalman Filter

Most physical systems are represented as continuous-time models while discrete-time measurements are frequently taken for state estimation via a digital processor. Therefore, the system model and measurement model are given by

\begin{align} \dot{\mathbf{x}}(t) &= f\bigl(\mathbf{x}(t), \mathbf{u}(t)\bigr) + \mathbf{w}(t), &\mathbf{w}(t) &\sim N\bigl(\mathbf{0},\mathbf{Q}(t)\bigr) \\ \mathbf{z}_k &= h(\mathbf{x}_k) + \mathbf{v}_k, &\mathbf{v}_k &\sim N(\mathbf{0},\mathbf{R}_k) \end{align}

where .

Initialize

\hat{\mathbf{x}}_{0|0}=E\bigl, \mathbf{P}_{0|0}=Var\bigl

Predict

\begin{align} &\begin{cases} \dot{\hat{\mathbf{x}}}(t) = f\bigl(\hat{\mathbf{x}}(t), \mathbf{u}(t)\bigr), \\ \dot{\mathbf{P}}(t) = \mathbf{F}(t)\mathbf{P}(t)+\mathbf{P}(t)\mathbf{F}(t)^\top + \mathbf{Q}(t), \end{cases}\qquad \text{with } \begin{cases} \hat{\mathbf{x}}(t_{k-1}) = \hat{\mathbf{x}}_{k-1|k-1}, \\ \mathbf{P}(t_{k-1}) = \mathbf{P}_{k-1|k-1}, \end{cases} \\ \Rightarrow &\begin{cases} \hat{\mathbf{x}}_{k|k-1} = \hat{\mathbf{x}}(t_k) \\ \mathbf{P}_{k|k-1} = \mathbf{P}(t_k) \end{cases} \end{align}

where

Update

where

The update equations are identical to those of discrete-time extended Kalman filter.

Read more about this topic:  Extended Kalman Filter

Famous quotes containing the word extended:

    “No: until I want the protection of Massachusetts to be extended to me in some distant Southern port, where my liberty is endangered, or until I am bent solely on building up an estate at home by peaceful enterprise, I can afford to refuse allegiance to Massachusetts, and her right to my property and life. It costs me less in every sense to incur the penalty of disobedience to the State than it would to obey. I should feel as if I were worth less in that case.”
    —Henry David Thoreau (1817–1862)