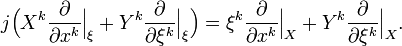Double Tangent Bundle - Secondary Vector Bundle Structure and Canonical Flip | Secondary Vector Bundle Structure Canonical Flip

Secondary Vector Bundle Structure and Canonical Flip

Since (TM,π_TM,M) is a vector bundle on its own right, its tangent bundle has the secondary vector bundle structure (TTM,(π_TM)_*,TM), where (π_TM)_*:TTM→TM is the push-forward of the canonical projection π_TM:TM→M. In the following we denote

$\xi = \xi^k\frac{\partial}{\partial x^k}\Big|_x\in T_xM, \qquad X = X^k\frac{\partial}{\partial x^k}\Big|_x\in T_xM$

and apply the associated coordinate system

$\xi \mapsto (x^1,\ldots,x^n,\xi^1,\ldots,\xi^n)$

on TM. Then the fibre of the secondary vector bundle structure at X∈T_xM takes the form

$(\pi_{TM})^{-1}_*(X) = \Big\{ \ X^k\frac{\partial}{\partial x^k}\Big|_\xi + Y^k\frac{\partial}{\partial\xi^k}\Big|_\xi \ \Big| \ \xi\in T_xM \, \ Y^1,\ldots,Y^n\in\R \ \Big\}.$

The canonical flip is a smooth involution j:TTM→TTM that exchanges these vector space structures in the sense that it is a vector bundle isomorphism between (TTM,π_TTM,TM) and (TTM,(π_TM)_*,TM). In the associated coordinates on TM it reads as

$j\Big(X^k\frac{\partial}{\partial x^k}\Big|_\xi + Y^k\frac{\partial}{\partial \xi^k}\Big|_\xi\Big) = \xi^k\frac{\partial}{\partial x^k}\Big|_X + Y^k\frac{\partial}{\partial \xi^k}\Big|_X.$

The canonical flip has the property that for any f: R2 → M,

$\frac {\partial f} {{\partial t} {\partial s}} = j \circ \frac {\partial f} {{\partial s} {\partial t}}$

where s and t are coordinates of the standard basis of R 2. Note that both partial derivatives are functions from R2 to TTM.

This property can, in fact, be used to give an intrinsic definition of the canonical flip. Indeed, there is a submersion p: J2₀ (R2,M) → TTM given by

$p=\frac {\partial f} {{\partial t} {\partial s}} (0,0)$

where p can be defined in the space of two-jets at zero because only depends on f up to order two at zero. We consider the application:

$J: J^2_0(\mathbb{R}^2,M) \to J^2_0(\mathbb{R}^2,M) \quad / \quad J=$

where α(s,t)= (t,s). Then J is compatible with the projection p and induces the canonical flip on the quotient TTM.

Read more about this topic: Double Tangent Bundle

Famous quotes containing the words secondary, bundle, structure, canonical and/or flip:

“Readers are less and less seen as mere non-writers, the subhuman “other” or flawed derivative of the author; the lack of a pen is no longer a shameful mark of secondary status but a positively enabling space, just as within every writer can be seen to lurk, as a repressed but contaminating antithesis, a reader.”
—Terry Eagleton (b. 1943)

“In the quilts I had found good objects—hospitable, warm, with soft edges yet resistant, with boundaries yet suggesting a continuous safe expanse, a field that could be bundled, a bundle that could be unfurled, portable equipment, light, washable, long-lasting, colorful, versatile, functional and ornamental, private and universal, mine and thine.”
—Radka Donnell-Vogt, U.S. quiltmaker. As quoted in Lives and Works, by Lynn F. Miller and Sally S. Swenson (1981)

“The structure was designed by an old sea captain who believed that the world would end in a flood. He built a home in the traditional shape of the Ark, inverted, with the roof forming the hull of the proposed vessel. The builder expected that the deluge would cause the house to topple and then reverse itself, floating away on its roof until it should land on some new Ararat.”
—For the State of New Jersey, U.S. public relief program (1935-1943)

“If God bestowed immortality on every man then when he made him, and he made many to whom he never purposed to give his saving grace, what did his Lordship think that God gave any man immortality with purpose only to make him capable of immortal torments? It is a hard saying, and I think cannot piously be believed. I am sure it can never be proved by the canonical Scripture.”
—Thomas Hobbes (1579–1688)

“By act of Congress, male officers are gentlemen, but by act of God, we are ladies. We don’t have to be little mini-men and try to be masculine and use obscene language to come across. I can take you and flip you on the floor and put your arms behind your back and you’ll never move again, without your ever knowing that I can do it.”
—Sherian Grace Cadoria (b. 1940)