Axial Multipole Moments - General Axial Multipole Moments

General Axial Multipole Moments

To get the general axial multipole moments, we replace the point charge of the previous section with an infinitesimal charge element, where represents the charge density at position on the z-axis. If the radius r of the observation point P is greater than the largest for which is significant (denoted ), the electric potential may be written

$\Phi(\mathbf{r}) = \frac{1}{4\pi\varepsilon} \sum_{k=0}^{\infty} M_{k} \left( \frac{1}{r^{k+1}} \right) P_{k}(\cos \theta )$

where the axial multipole moments are defined

$M_{k} \equiv \int d\zeta \ \lambda(\zeta) \zeta^{k}$

Special cases include the axial monopole moment (=total charge)

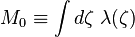$M_{0} \equiv \int d\zeta \ \lambda(\zeta)$ ,

the axial dipole moment, and the axial quadrupole moment . Each successive term in the expansion varies inversely with a greater power of, e.g., the monopole potential varies as, the dipole potential varies as, the quadrupole potential varies as, etc. Thus, at large distances, the potential is well-approximated by the leading nonzero multipole term.

The lowest non-zero axial multipole moment is invariant under a shift b in origin, but higher moments generally depend on the choice of origin. The shifted multipole moments would be

$M_{k}^{\prime} \equiv \int d\zeta \ \lambda(\zeta) \ \left(\zeta + b \right)^{k}$

Expanding the polynomial under the integral

$\left( \zeta + b \right)^{l} = \zeta^{l} + l b \zeta^{l-1} + \ldots + l \zeta b^{l-1} + b^{l}$

leads to the equation

$M_{k}^{\prime} = M_{k} + l b M_{k-1} + \ldots + l b^{l-1} M_{1} + b^{l} M_{0}$

If the lower moments are zero, then . The same equation shows that multipole moments higher than the first non-zero moment do depend on the choice of origin (in general).

Read more about this topic: Axial Multipole Moments

Famous quotes containing the words general and/or moments:

“In communist society, where nobody has one exclusive sphere of activity but each can become accomplished in any branch he wishes, society regulates the general production and thus makes it possible for me to do one thing today and another tomorrow, to hunt in the morning, fish in the afternoon, rear cattle in the evening, criticize after dinner, just as I have a mind, without ever becoming hunter, fisherman, shepherd or critic.”
—Karl Marx (1818–1883)

“The government does not concern me much, and I shall bestow the fewest possible thoughts on it. It is not many moments that I live under a government, even in this world. If a man is thought- free, fancy-free, imagination-free ... unwise rulers or reformers cannot fatally interrupt him.”
—Henry David Thoreau (1817–1862)