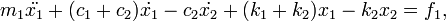Vibration - Multiple Degrees of Freedom Systems and Mode Shapes

Multiple Degrees of Freedom Systems and Mode Shapes

The simple mass–spring damper model is the foundation of vibration analysis, but what about more complex systems? The mass–spring–damper model described above is called a single degree of freedom (SDOF) model since we have assumed the mass only moves up and down. In the case of more complex systems we need to discretize the system into more masses and allow them to move in more than one direction – adding degrees of freedom. The major concepts of multiple degrees of freedom (MDOF) can be understood by looking at just a 2 degree of freedom model as shown in the figure.

The equations of motion of the 2DOF system are found to be:

m_1 \ddot{x_1} + { (c_1+c_2) } \dot{x_1} - { c_2 } \dot{x_2}+ { (k_1+k_2) } x_1 -{ k_2 } x_2= f_1,
m_2 \ddot{x_2} - { c_2 } \dot{x_1}+ { (c_2+c_3) } \dot{x_2} - { k_2 } x_1+ { (k_2+k_3) } x_2 = f_2. \!

We can rewrite this in matrix format:

\begin{bmatrix}m_1 & 0\\ 0 & m_2\end{bmatrix}\begin{Bmatrix}\ddot{x_1}\\ \ddot{x_2}\end{Bmatrix}+\begin{bmatrix}c_1+c_2 & -c_2\\ -c_2 & c_2+c_3\end{bmatrix}\begin{Bmatrix}\dot{x_1}\\ \dot{x_2}\end{Bmatrix}+\begin{bmatrix}k_1+k_2 & -k_2\\ -k_2 & k_2+k_3\end{bmatrix}\begin{Bmatrix} x_1\\ x_2\end{Bmatrix}=\begin{Bmatrix} f_1\\ f_2\end{Bmatrix}.

A more compact form of this matrix equation can be written as:

\begin{bmatrix}M\end{bmatrix}\begin{Bmatrix}\ddot{x}\end{Bmatrix}+\begin{bmatrix}C\end{bmatrix}\begin{Bmatrix}\dot{x}\end{Bmatrix}+\begin{bmatrix}K\end{bmatrix}\begin{Bmatrix} x\end{Bmatrix}=\begin{Bmatrix} f \end{Bmatrix}

where and are symmetric matrices referred respectively as the mass, damping, and stiffness matrices. The matrices are NxN square matrices where N is the number of degrees of freedom of the system.

In the following analysis we will consider the case where there is no damping and no applied forces (i.e. free vibration). The solution of a viscously damped system is somewhat more complicated.

This differential equation can be solved by assuming the following type of solution:

\begin{Bmatrix} x\end{Bmatrix}=\begin{Bmatrix} X\end{Bmatrix}e^{i\omega t}.

Note: Using the exponential solution of is a mathematical trick used to solve linear differential equations. If we use Euler's formula and take only the real part of the solution it is the same cosine solution for the 1 DOF system. The exponential solution is only used because it easier to manipulate mathematically.

The equation then becomes:

Since cannot equal zero the equation reduces to the following.

Read more about this topic:  Vibration

Famous quotes containing the words multiple, degrees, freedom, systems, mode and/or shapes:

    “Creativity seems to emerge from multiple experiences, coupled with a well-supported development of personal resources, including a sense of freedom to venture beyond the known.”
    —Loris Malaguzzi (20th century)

    “The most durable thing in writing is style, and style is the most valuable investment a writer can make with his time. It pays off slowly, your agent will sneer at it, your publisher will misunderstand it, and it will take people you have never heard of to convince them by slow degrees that the writer who puts his individual mark on the way he writes will always pay off.”
    —Raymond Chandler (1888–1959)

    “Old-fashioned determinism was what we may call hard determinism. It did not shrink from such words as fatality, bondage of the will, necessitation, and the like. Nowadays, we have a soft determinism which abhors harsh words, and, repudiating fatality, necessity, and even predetermination, says that its real name is freedom; for freedom is only necessity understood, and bondage to the highest is identical with true freedom.”
    —William James (1842–1910)

    “What avails it that you are a Christian, if you are not purer than the heathen, if you deny yourself no more, if you are not more religious? I know of many systems of religion esteemed heathenish whose precepts fill the reader with shame, and provoke him to new endeavors, though it be to the performance of rites merely.”
    —Henry David Thoreau (1817–1862)

    “In most cases a favorite writer is more with us in his book than he ever could have been in the flesh; since, being a writer, he is one who has studied and perfected this particular mode of personal incarnation, very likely to the detriment of any other. I should like as a matter of curiosity to see and hear for a moment the men whose works I admire; but I should hardly expect to find further intercourse particularly profitable.”
    —Charles Horton Cooley (1864–1929)

    “No, no; but as in my idolatry
    I said to all my profane mistresses,
    Beauty, of pity, foulness only is
    A sign of rigour: so I say to thee,
    To wicked spirits are horrid shapes assign’d,
    This beauteous form assures a piteous mind.”
    —John Donne (1572–1631)