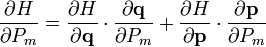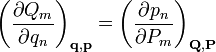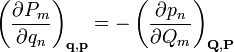Canonical Transformation - Direct Approach

Direct Approach

The functional form of Hamilton's equations is

$\dot{\mathbf{p}} = -\frac{\partial H}{\partial \mathbf{q}}$

$\dot{\mathbf{q}} =~~\frac{\partial H}{\partial \mathbf{p}}$

By definition, the transformed coordinates have analogous dynamics

$\dot{\mathbf{P}} = -\frac{\partial K}{\partial \mathbf{Q}}$

$\dot{\mathbf{Q}} =~~\frac{\partial K}{\partial \mathbf{P}}$

where K(Q,P) is a new Hamiltonian that must be determined.

In general, a transformation (q,p,t) → (Q,P,t) does not preserve the form of Hamilton's equations. For time independent transformations between (q,p) and (Q,P) we may check if the transformation is restricted canonical, as follows. Since restricted transformations have no explicit time dependence (by construction), the time derivative of a new generalized coordinate Q_m is

$\dot{Q}_{m} = \frac{\partial Q_{m}}{\partial \mathbf{q}} \cdot \dot{\mathbf{q}} + \frac{\partial Q_{m}}{\partial \mathbf{p}} \cdot \dot{\mathbf{p}} = \frac{\partial Q_{m}}{\partial \mathbf{q}} \cdot \frac{\partial H}{\partial \mathbf{p}} - \frac{\partial Q_{m}}{\partial \mathbf{p}} \cdot \frac{\partial H}{\partial \mathbf{q}} = \lbrace Q_m, H \rbrace$

where is the Poisson bracket.

We also have the identity for the conjugate momentum P_m

$\frac{\partial H}{\partial P_{m}} = \frac{\partial H}{\partial \mathbf{q}} \cdot \frac{\partial \mathbf{q}}{\partial P_{m}} + \frac{\partial H}{\partial \mathbf{p}} \cdot \frac{\partial \mathbf{p}}{\partial P_{m}}$

If the transformation is canonical, these two must be equal, resulting in the equations

$\left( \frac{\partial Q_{m}}{\partial p_{n}}\right)_{\mathbf{q}, \mathbf{p}} = -\left( \frac{\partial q_{n}}{\partial P_{m}}\right)_{\mathbf{Q}, \mathbf{P}}$

$\left( \frac{\partial Q_{m}}{\partial q_{n}}\right)_{\mathbf{q}, \mathbf{p}} = \left( \frac{\partial p_{n}}{\partial P_{m}}\right)_{\mathbf{Q}, \mathbf{P}}$

The analogous argument for the generalized momenta P_m leads to two other sets of equations

$\left( \frac{\partial P_{m}}{\partial p_{n}}\right)_{\mathbf{q}, \mathbf{p}} = \left( \frac{\partial q_{n}}{\partial Q_{m}}\right)_{\mathbf{Q}, \mathbf{P}}$

$\left( \frac{\partial P_{m}}{\partial q_{n}}\right)_{\mathbf{q}, \mathbf{p}} = -\left( \frac{\partial p_{n}}{\partial Q_{m}}\right)_{\mathbf{Q}, \mathbf{P}}$

These are the direct conditions to check whether a given transformation is canonical.

Read more about this topic: Canonical Transformation

Famous quotes containing the words direct and/or approach:

“James’s great gift, of course, was his ability to tell a plot in shimmering detail with such delicacy of treatment and such fine aloofness—that is, reluctance to engage in any direct grappling with what, in the play or story, had actually “taken place”Mthat his listeners often did not, in the end, know what had, to put it in another way, “gone on.””
—James Thurber (1894–1961)

“Genuine polemics approach a book as lovingly as a cannibal spices a baby.”
—Walter Benjamin (1892–1940)