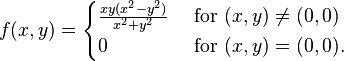Non-symmetry
In the worst case symmetry fails. Given two variables near (0, 0) and two limiting processes on
corresponding to making h → 0 first, and to making k → 0 first. These processes need not commute (see interchange of limiting operations): it can matter, looking at the first-order terms, which is applied first. This leads to the construction of pathological examples in which second derivatives are non-symmetric. Given that the derivatives as Schwartz distributions are symmetric, this kind of example belongs in the 'fine' theory of real analysis.
The following example displays non-symmetry. Note that it does not violate Clairaut's theorem since the derivatives are not continuous at (0,0)
The mixed partial derivatives of f exist and are continuous everywhere except at . Moreover
at .
Read more about this topic: Symmetry Of Second Derivatives