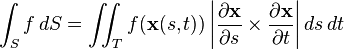Surface Integral - Surface Integrals of Scalar Fields

Surface Integrals of Scalar Fields

To find an explicit formula for the surface integral, we need to parameterize the surface of interest, S, by considering a system of curvilinear coordinates on S, like the latitude and longitude on a sphere. Let such a parameterization be x(s, t), where (s, t) varies in some region T in the plane. Then, the surface integral is given by

\int_{S} f \,dS = \iint_{T} f(\mathbf{x}(s, t)) \left|{\partial \mathbf{x} \over \partial s}\times {\partial \mathbf{x} \over \partial t}\right| ds\, dt

where the expression between bars on the right-hand side is the magnitude of the cross product of the partial derivatives of x(s, t), and is known as the surface element.

For example, if we want to find the surface area of some general scalar function, say, we have

A = \int_S \,dS = \iint_T \left\|{\partial \mathbf{r} \over \partial x}\times {\partial \mathbf{r} \over \partial y}\right\| dx\, dy

where . So that, and . So,

\begin{align} A &{} = \iint_T \left\|\left(1, 0, {\partial f \over \partial x}\right)\times \left(0, 1, {\partial f \over \partial y}\right)\right\| dx\, dy \\ &{} = \iint_T \left\|\left(-{\partial f \over \partial x}, -{\partial f \over \partial y}, 1\right)\right\| dx\, dy \\ &{} = \iint_T \sqrt{\left({\partial f \over \partial x}\right)^2+\left({\partial f \over \partial y}\right)^2+1}\, \, dx\, dy \end{align}

which is the familiar formula we get for the surface area of a general functional shape. One can recognize the vector in the second line above as the normal vector to the surface.

Note that because of the presence of the cross product, the above formulas only work for surfaces embedded in three-dimensional space.

Read more about this topic:  Surface Integral

Famous quotes containing the words surface and/or fields:

    “I cannot but conclude the bulk of your natives to be the most pernicious race of little, odious vermin that Nature ever suffered to crawl upon the surface of the earth.”
    —Jonathan Swift (1667–1745)

    “If the sight of the blue skies fills you with joy, if a blade of grass springing up in the fields has power to move you, if the simple things of nature have a message that you understand, rejoice, for your soul is alive ...”
    —Eleonora Duse (1859–1924)