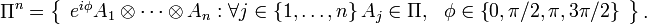Stabilizer Code - Mathematical Background

Mathematical Background

The Stabilizer formalism exploits elements of the Pauli group in formulating quantum error-correcting codes. The set consists of the Pauli operators:

I\equiv \begin{bmatrix} 1 & 0\\ 0 & 1 \end{bmatrix} ,\ X\equiv \begin{bmatrix} 0 & 1\\ 1 & 0 \end{bmatrix} ,\ Y\equiv \begin{bmatrix} 0 & -i\\ i & 0 \end{bmatrix} ,\ Z\equiv \begin{bmatrix} 1 & 0\\ 0 & -1 \end{bmatrix} .

The above operators act on a single qubit---a state represented by a vector in a two-dimensional Hilbert space. Operators in have eigenvalues and either commute or anti-commute. The set consists of -fold tensor products of Pauli operators:

\Pi^{n}=\left\{ \begin{array} {c} e^{i\phi}A_{1}\otimes\cdots\otimes A_{n}:\forall j\in\left\{ 1,\ldots ,n\right\} A_{j}\in\Pi,\ \ \phi\in\left\{ 0,\pi/2,\pi,3\pi/2\right\} \end{array} \right\} .

Elements of act on a quantum register of qubits. We occasionally omit tensor product symbols in what follows so that

The -fold Pauli group plays an important role for both the encoding circuit and the error-correction procedure of a quantum stabilizer code over qubits.

Read more about this topic:  Stabilizer Code

Famous quotes containing the words mathematical and/or background:

    “All science requires mathematics. The knowledge of mathematical things is almost innate in us.... This is the easiest of sciences, a fact which is obvious in that no one’s brain rejects it; for laymen and people who are utterly illiterate know how to count and reckon.”
    —Roger Bacon (c. 1214–c. 1294)

    “In the true sense one’s native land, with its background of tradition, early impressions, reminiscences and other things dear to one, is not enough to make sensitive human beings feel at home.”
    —Emma Goldman (1869–1940)