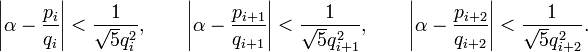Square Root of 5 - Diophantine Approximations

Diophantine Approximations

Hurwitz's theorem in Diophantine approximations states that every irrational number x can be approximated by infinitely many rational numbers m/n in lowest terms in such a way that

and that √5 is best possible, in the sense that for any larger constant than √5, there are some irrational numbers x for which only finitely many such approximations exist.

Closely related to this is the theorem that of any three consecutive convergents p_i/q_i, p_i+1/q_i+1, p_i+2/q_i+2, of a number α, at least one of the three inequalities holds:

$\left|\alpha - {p_i\over q_i}\right| < {1\over \sqrt5 q_i^2}, \qquad \left|\alpha - {p_{i+1}\over q_{i+1}}\right| < {1\over \sqrt5 q_{i+1}^2}, \qquad \left|\alpha - {p_{i+2}\over q_{i+2}}\right| < {1\over \sqrt5 q_{i+2}^2}.$

And the √5 in the denominator is the best bound possible since the convergents of the golden ratio make the difference on the left-hand side arbitrarily close to the value on the right-hand side. In particular, one cannot obtain a tighter bound by considering sequences of four or more consecutive convergents.

Read more about this topic: Square Root Of 5