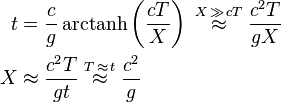Rindler Coordinates - Relation To Cartesian Chart

Relation To Cartesian Chart

To obtain the Rindler chart, start with the Cartesian chart (an inertial frame) with the metric

In the region, which is often called the Rindler wedge, if g represents the proper acceleration (along the hyperbola x=1) of the Rindler observer whose proper time is defined to be equal to Rindler coordinate time (see below), the new chart is defined using the coordinate transformation

The inverse transformation is

In the Rindler chart, the Minkowski line element becomes

Any observer at rest in Rindler coordinates has constant proper acceleration, with Rindler observers closer to the Rindler horizon having greater proper acceleration. All the Rindler observers are instantaneously at rest at time T=0 in the inertial frame, and at this time a Rindler observer with proper acceleration gi will be at position X = 1/gi (really X = c2/gi, but we assume units where c=1), which is also that observer's constant distance from the Rindler horizon in Rindler coordinates. If all Rindler observers set their clocks to zero at T=0, then when defining a Rindler coordinate system we have a choice of which Rindler observer's proper time will be equal to the coordinate time t in Rindler coordinates, and this observer's proper acceleration defines the value of g above (for other Rindler observers at different distances from the Rindler horizon, the coordinate time will equal some constant multiple of their own proper time). It is a common convention to define the Rindler coordinate system so that the Rindler observer whose proper time matches coordinate time is the one who has proper acceleration g=1, so that g can be eliminated from the equations

The above equation,

has been simplified for c=1. The unsimplified equation is more convenient for finding the Rindler Horizon distance, given an acceleration g.

\begin{align} t &= \frac{c}{g} \operatorname{arctanh}\left (\frac{c T}{X}\right) \;\overset {X \,\gg\, cT}\approx\; \frac{c^2 T}{g X}\\ X &\approx \frac{c^2 T}{g t} \;\overset{T \,\approx\, t}\approx\; \frac{c^2 }{g} \end{align}


The remainder of the article will follow the convention of setting both g=1 and c=1, so units for X and x will be 1 unit = c^2/g = 1. Be mindful that setting g=1 light-second/second2 is very different from setting g=1 light-year/year^2. Even if we pick units where c=1, the magnitude of the proper acceleration g will depend on our choice of units: for example, if we use units of light-years for distance, (X or x) and years for time, (T or t), this would mean g = 1 light year/year2, equal to about 9.5 meters/second2, while if we use units of light-seconds for distance, (X or x), and seconds for time, (T or t), this would mean g = 1 light-second/second2, or 299792458 meters/second2).

Read more about this topic:  Rindler Coordinates

Famous quotes containing the words relation to, relation and/or chart:

    “A theory of the middle class: that it is not to be determined by its financial situation but rather by its relation to government. That is, one could shade down from an actual ruling or governing class to a class hopelessly out of relation to government, thinking of gov’t as beyond its control, of itself as wholly controlled by gov’t. Somewhere in between and in gradations is the group that has the sense that gov’t exists for it, and shapes its consciousness accordingly.”
    —Lionel Trilling (1905–1975)

    “Only in a house where one has learnt to be lonely does one have this solicitude for things. One’s relation to them, the daily seeing or touching, begins to become love, and to lay one open to pain.”
    —Elizabeth Bowen (1899–1973)

    “Perhaps in His wisdom the Almighty is trying to show us that a leader may chart the way, may point out the road to lasting peace, but that many leaders and many peoples must do the building.”
    —Eleanor Roosevelt (1884–1962)