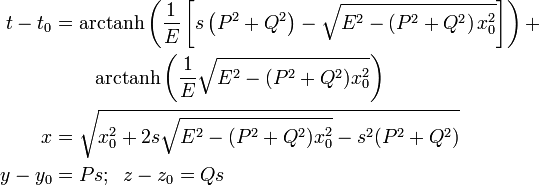Rindler Coordinates - Geodesics

Geodesics

The geodesic equations in the Rindler chart are easily obtained from the geodesic Lagrangian; they are

Of course, in the original Cartesian chart, the geodesics appear as straight lines, so we could easily obtain them in the Rindler chart using our coordinate transformation. However, it is instructive to obtain and study them independently of the original chart, and we shall do so in this section.

From the first, third, and fourth we immediately obtain the first integrals

But from the line element we have where for timelike, null, and spacelike geodesics, respectively. This gives the fourth first integral, namely

.

This suffices to give the complete solution of the geodesic equations.

In the case of null geodesics, from with nonzero, we see that the x coordinate ranges over the interval .

The complete seven parameter family giving any null geodesic through any event in the Rindler wedge, is

Plotting the tracks of some representative null geodesics through a given event (that is, projecting to the hyperslice ), we obtain a picture which looks suspiciously like the family of all semicircles through a point and orthogonal to the Rindler horizon! (See the figure.)