Renormalization Group - Block Spin Renormalization Group

Block Spin Renormalization Group

This section introduces pedagogically a picture of RG which may be easiest to grasp: the block spin RG. It was devised by Leo P. Kadanoff in 1966.

Let us consider a 2D solid, a set of atoms in a perfect square array, as depicted in the figure. Let us assume that atoms interact among themselves only with their nearest neighbours, and that the system is at a given temperature . The strength of their interaction is measured by a certain coupling constant . The physics of the system will be described by a certain formula, say .

Now we proceed to divide the solid into blocks of 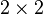squares; we attempt to describe the system in terms of block variables, i.e.: some variables which describe the average behavior of the block. Also, let us assume that, due to a lucky coincidence, the physics of block variables is described by a formula of the same kind, but with different values for and : . (This isn't exactly true, of course, but it is often approximately true in practice, and that is good enough, to a first approximation.)

Perhaps the initial problem was too hard to solve, since there were too many atoms. Now, in the renormalized problem we have only one fourth of them. But why should we stop now? Another iteration of the same kind leads to, and only one sixteenth of the atoms. We are increasing the observation scale with each RG step.

Of course, the best idea is to iterate until there is only one very big block. Since the number of atoms in any real sample of material is very large, this is more or less equivalent to finding the long term behaviour of the RG transformation which took and . Usually, when iterated many times, this RG transformation leads to a certain number of fixed points.

Let us be more concrete and consider a magnetic system (e.g.: the Ising model), in which the J coupling constant denotes the trend of neighbour spins to be parallel. The configuration of the system is the result of the tradeoff between the ordering J term and the disordering effect of temperature. For many models of this kind there are three fixed points:

1. and . This means that, at the largest size, temperature becomes unimportant, i.e.: the disordering factor vanishes. Thus, in large scales, the system appears to be ordered. We are in a ferromagnetic phase.
2. and . Exactly the opposite, temperature dominates, and the system is disordered at large scales.
3. A nontrivial point between them, and . In this point, changing the scale does not change the physics, because the system is in a fractal state. It corresponds to the Curie phase transition, and is also called a critical point.

So, if we are given a certain material with given values of T and J, all we have to do in order to find out the large scale behaviour of the system is to iterate the pair until we find the corresponding fixed point.