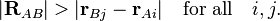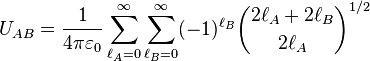Multipole Expansion - Interaction of Two Non-overlapping Charge Distributions

Interaction of Two Non-overlapping Charge Distributions

Consider two sets of point charges, one set {qi } clustered around a point A and one set {qj } clustered around a point B. Think for example of two molecules, and recall that a molecule by definition consists of electrons (negative point charges) and nuclei (positive point charges). The total electrostatic interaction energy UAB between the two distributions is

U_{AB} = \sum_{i\in A} \sum_{j\in B} \frac{q_i q_j}{4\pi\varepsilon_0 r_{ij}}.

This energy can be expanded in a power series in the inverse distance of A and B. This expansion is known as the multipole expansion of UAB.

In order to derive this multipole expansion, we write rXY = rY-rX, which is a vector pointing from X towards Y. Note that

\mathbf{R}_{AB}+\mathbf{r}_{Bj}+\mathbf{r}_{ji}-\mathbf{r}_{iA} = 0 \quad\Leftrightarrow\quad \mathbf{r}_{ij} = \mathbf{R}_{AB}-\mathbf{r}_{Ai}+\mathbf{r}_{Bj} .

We assume that the two distributions do not overlap:

|\mathbf{R}_{AB}| > |\mathbf{r}_{Bj}-\mathbf{r}_{Ai}| \quad\hbox{for all}\quad i,j.

Under this condition we may apply the Laplace expansion in the following form

\frac{1}{|\mathbf{r}_{j}-\mathbf{r}_i|} = \frac{1}{|\mathbf{R}_{AB} - (\mathbf{r}_{Ai}- \mathbf{r}_{Bj})| } = \sum_{L=0}^\infty \sum_{M=-L}^L \, (-1)^M I_L^{-M}(\mathbf{R}_{AB})\; R^M_{L}( \mathbf{r}_{Ai}-\mathbf{r}_{Bj}),

where and are irregular and regular solid harmonics, respectively. The translation of the regular solid harmonic gives a finite expansion,

R^M_L(\mathbf{r}_{Ai}-\mathbf{r}_{Bj}) = \sum_{\ell_A=0}^L (-1)^{L-\ell_A} \binom{2L}{2\ell_A}^{1/2}
\times \sum_{m_A=-\ell_A}^{\ell_A} R^{m_A}_{\ell_A}(\mathbf{r}_{Ai}) R^{M-m_A}_{L-\ell_A}(\mathbf{r}_{Bj})\; \langle \ell_A, m_A; L-\ell_A, M-m_A| L M \rangle,

where the quantity between pointed brackets is a Clebsch-Gordan coefficient. Further we used

R^{m}_{\ell}(-\mathbf{r}) = (-1)^{\ell} R^{m}_{\ell}(\mathbf{r}) .

Use of the definition of spherical multipoles Qml and covering of the summation ranges in a somewhat different order (which is only allowed for an infinite range of L) gives finally

U_{AB} = \frac{1}{4\pi\varepsilon_0} \sum_{\ell_A=0}^\infty \sum_{\ell_B=0}^\infty (-1)^{\ell_B} \binom{2\ell_A+2\ell_B}{2\ell_A}^{1/2} \,
\times \sum_{m_A=-\ell_A}^{\ell_A} \sum_{m_B=-\ell_B}^{\ell_B}(-1)^{m_A+m_B} I_{\ell_A+\ell_B}^{-m_A-m_B}(\mathbf{R}_{AB})\; Q^{m_A}_{\ell_A} Q^{m_B}_{\ell_B}\; \langle \ell_A, m_A; \ell_B, m_B| \ell_A+\ell_B, m_A+m_B \rangle.

This is the multipole expansion of the interaction energy of two non-overlapping charge distributions which are a distance RAB apart. Since

I_{\ell_A+\ell_B}^{-(m_A+m_B)}(\mathbf{R}_{AB}) \equiv \left^{1/2}\; \frac{Y^{-(m_A+m_B)}_{\ell_A+\ell_B}(\widehat{\mathbf{R}}_{AB})}{R^{\ell_A+\ell_B+1}_{AB}}

this expansion is manifestly in powers of 1/RAB. The function Yml is a normalized spherical harmonic.

Read more about this topic:  Multipole Expansion

Famous quotes containing the words interaction of, interaction and/or charge:

    “Here is this vast, savage, howling mother of ours, Nature, lying all around, with such beauty, and such affection for her children, as the leopard; and yet we are so early weaned from her breast to society, to that culture which is exclusively an interaction of man on man,—a sort of breeding in and in, which produces at most a merely English nobility, a civilization destined to have a speedy limit.”
    —Henry David Thoreau (1817–1862)

    “Here is this vast, savage, howling mother of ours, Nature, lying all around, with such beauty, and such affection for her children, as the leopard; and yet we are so early weaned from her breast to society, to that culture which is exclusively an interaction of man on man,—a sort of breeding in and in, which produces at most a merely English nobility, a civilization destined to have a speedy limit.”
    —Henry David Thoreau (1817–1862)

    “Those things for which the most money is demanded are never the things which the student most wants. Tuition, for instance, is an important item in the term bill, while for the far more valuable education which he gets by associating with the most cultivated of his contemporaries no charge is made.”
    —Henry David Thoreau (1817–1862)