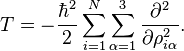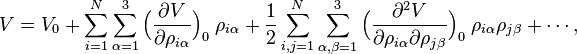Molecular Hamiltonian - Harmonic Nuclear Motion Hamiltonian

Harmonic Nuclear Motion Hamiltonian

In the remaining part of this article we assume that the molecule is semi-rigid. In the second step of the BO approximation the nuclear kinetic energy T_n is reintroduced and the Schrödinger equation with Hamiltonian

$\hat{H}_\mathrm{nuc} = -\frac{\hbar^2}{2}\sum_{i=1}^N \sum_{\alpha=1}^3 \frac{1}{M_i} \frac{\partial^2}{\partial R_{i\alpha}^2} +V(\mathbf{R}_1,\ldots,\mathbf{R}_N)$

is considered. One would like to recognize in its solution: the motion of the nuclear center of mass (3 degrees of freedom), the overall rotation of the molecule (3 degrees of freedom), and the nuclear vibrations. In general, this is not possible with the given nuclear kinetic energy, because it does not separate explicitly the 6 external degrees of freedom (overall translation and rotation) from the 3N − 6 internal degrees of freedom. In fact, the kinetic energy operator here is defined with respect to a space-fixed (SF) frame. If we were to move the origin of the SF frame to the nuclear center of mass, then, by application of the chain rule, nuclear mass polarization terms would appear. It is customary to ignore these terms altogether and we will follow this custom.

In order to achieve a separation we must distinguish internal and external coordinates, to which end Eckart introduced conditions to be satisfied by the coordinates. We will show how these conditions arise in a natural way from a harmonic analysis in mass-weighted Cartesian coordinates.

In order to simplify the expression for the kinetic energy we introduce mass-weighted displacement coordinates

Since

$\frac{\partial}{\partial \rho_{i \alpha}} = \frac{\partial}{\sqrt{M_i} (\partial R_{i \alpha} - \partial R^0_{i \alpha})} = \frac{1}{\sqrt{M_i}} \frac{\partial}{\partial R_{i \alpha}} ,$

the kinetic energy operator becomes,

$T = -\frac{\hbar^2}{2} \sum_{i=1}^N \sum_{\alpha=1}^3 \frac{\partial^2}{\partial \rho_{i\alpha}^2}.$

If we make a Taylor expansion of V around the equilibrium geometry,

$V = V_0 + \sum_{i=1}^N \sum_{\alpha=1}^3 \Big(\frac{\partial V}{\partial \rho_{i\alpha}}\Big)_0\; \rho_{i\alpha} + \frac{1}{2} \sum_{i,j=1}^N \sum_{\alpha,\beta=1}^3 \Big( \frac{\partial^2 V}{\partial \rho_{i\alpha}\partial\rho_{j\beta}}\Big)_0 \;\rho_{i\alpha}\rho_{j\beta} + \cdots,$

and truncate after three terms (the so-called harmonic approximation), we can describe V with only the third term. The term V₀ can be absorbed in the energy (gives a new zero of energy). The second term is vanishing because of the equilibrium condition. The remaining term contains the Hessian matrix F of V, which is symmetric and may be diagonalized with an orthogonal 3N × 3N matrix with constant elements:

$\mathbf{Q} \mathbf{F} \mathbf{Q}^\mathrm{T} = \boldsymbol{\Phi} \quad \mathrm{with}\quad \boldsymbol{\Phi} = \operatorname{diag}(f_1, \dots, f_{3N-6}, 0,\ldots,0).$

It can be shown from the invariance of V under rotation and translation that six of the eigenvectors of F (last six rows of Q) have eigenvalue zero (are zero-frequency modes). They span the external space. The first 3N − 6 rows of Q are—for molecules in their ground state—eigenvectors with non-zero eigenvalue; they are the internal coordinates and form an orthonormal basis for a (3N - 6)-dimensional subspace of the nuclear configuration space R3N, the internal space. The zero-frequency eigenvectors are orthogonal to the eigenvectors of non-zero frequency. It can be shown that these orthogonalities are in fact the Eckart conditions. The kinetic energy expressed in the internal coordinates is the internal (vibrational) kinetic energy.

With the introduction of normal coordinates

$q_t \equiv \sum_{i=1}^N\sum_{\alpha=1}^3 \; Q_{t, i\alpha} \rho_{i\alpha},$

the vibrational (internal) part of the Hamiltonian for the nuclear motion becomes in the harmonic approximation

$\hat{H}_\mathrm{nuc} \approx \frac{1}{2} \sum_{t=1}^{3N-6} \left .$

The corresponding Schrödinger equation is easily solved, it factorizes into 3N − 6 equations for one-dimensional harmonic oscillators. The main effort in this approximate solution of the nuclear motion Schrödinger equation is the computation of the Hessian F of V and its diagonalization.

This approximation to the nuclear motion problem, described in 3N mass-weighted Cartesian coordinates, became standard in quantum chemistry, since the days (1980s-1990s) that algorithms for accurate computations of the Hessian F became available. Apart from the harmonic approximation, it has as a further deficiency that the external (rotational and translational) motions of the molecule are not accounted for. They are accounted for in a rovibrational Hamiltonian that sometimes is called Watson's Hamiltonian.

Read more about this topic: Molecular Hamiltonian

Famous quotes containing the words harmonic, nuclear and/or motion:

“For decades child development experts have erroneously directed parents to sing with one voice, a unison chorus of values, politics, disciplinary and loving styles. But duets have greater harmonic possibilities and are more interesting to listen to, so long as cacophony or dissonance remains at acceptable levels.”
—Kyle D. Pruett (20th century)

“We now recognize that abuse and neglect may be as frequent in nuclear families as love, protection, and commitment are in nonnuclear families.”
—David Elkind (20th century)

“Too many Broadway actors in motion pictures lost their grip on success—had a feeling that none of it had ever happened on that sun-drenched coast, that the coast itself did not exist, there was no California. It had dropped away like a hasty dream and nothing could ever have been like the things they thought they remembered.”
—Mae West (1892–1980)