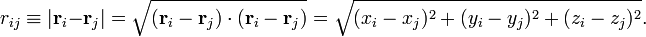Molecular Hamiltonian - Coulomb Hamiltonian

Coulomb Hamiltonian

The algebraic form of many observables—i.e., Hermitian operators representing observable quantities—is obtained by the following quantization rules:

Write the classical form of the observable in Hamilton form (as a function of momenta p and positions q). Both vectors are expressed with respect to an arbitrary inertial frame, usually referred to as laboratory-frame or space-fixed frame.
Replace p by and interpret q as a multiplicative operator. Here is the nabla operator, a vector operator consisting of first derivatives. The well-known commutation relations for the p and q operators follow directly from the differentiation rules.

Classically the electrons and nuclei in a molecule have kinetic energy of the form p2/(2m) and interact via Coulomb interactions, which are inversely proportional to the distance r_ij between particle i and j.

$r_{ij} \equiv |\mathbf{r}_i -\mathbf{r}_j| = \sqrt{(\mathbf{r}_i -\mathbf{r}_j)\cdot(\mathbf{r}_i -\mathbf{r}_j)} = \sqrt{(x_i-x_j)^2 + (y_i-y_j)^2 + (z_i-z_j)^2 } .$

In this expression r_i stands for the coordinate vector of any particle (electron or nucleus), but from here on we will reserve capital R to represent the nuclear coordinate, and lower case r for the electrons of the system. The coordinates can be taken to be expressed with respect to any Cartesian frame centered anywhere in space, because distance, being an inner product, is invariant under rotation of the frame and, being the norm of a difference vector, distance is invariant under translation of the frame as well.

By quantizing the classical energy in Hamilton form one obtains the a molecular Hamilton operator that is often referred to as the Coulomb Hamiltonian. This Hamiltonian is a sum of five terms. They are

The kinetic energy operators for each nucleus in the system;
The kinetic energy operators for each electron in the system;
The potential energy between the electrons and nuclei – the total electron-nucleus Coulombic attraction in the system;
The potential energy arising from Coulombic electron-electron repulsions
The potential energy arising from Coulombic nuclei-nuclei repulsions - also known as the nuclear repulsion energy. See electric potential for more details.

$\hat{U}_{ee} = {1 \over 2} \sum_i \sum_{j \ne i} \frac{e^2}{4 \pi \epsilon_0 \left | \mathbf{r}_i - \mathbf{r}_j \right | } = \sum_i \sum_{j > i} \frac{e^2}{4 \pi \epsilon_0 \left | \mathbf{r}_i - \mathbf{r}_j \right | }$
$\hat{U}_{nn} = {1 \over 2} \sum_i \sum_{j \ne i} \frac{Z_i Z_j e^2}{4 \pi \epsilon_0 \left | \mathbf{R}_i - \mathbf{R}_j \right | } = \sum_i \sum_{j > i} \frac{Z_i Z_j e^2}{4 \pi \epsilon_0 \left | \mathbf{R}_i - \mathbf{R}_j \right | }.$

Here M_i is the mass of nucleus i, Z_i is the atomic number of nucleus i, and m_e is the mass of the electron. The Laplace operator of particle i is : $\nabla^2_{\mathbf{r}_i} \equiv \boldsymbol{\nabla}_{\mathbf{r}_i}\cdot \boldsymbol{\nabla}_{\mathbf{r}_i} = \frac{\partial^2}{\partial x_i^2} + \frac{\partial^2}{\partial y_i^2} + \frac{\partial^2}{\partial z_i^2}$ . Since the kinetic energy operator is an inner product, it is invariant under rotation of the Cartesian frame with respect to which x_i, y_i, and z_i are expressed.

Read more about this topic: Molecular Hamiltonian