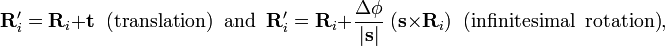Molecular Hamiltonian - Clamped Nucleus Hamiltonian

Clamped Nucleus Hamiltonian

The clamped nucleus Hamiltonian describes the energy of the electrons in the electrostatic field of the nuclei, where the nuclei are assumed to be stationary with respect to an inertial frame. The form of the electronic Hamiltonian is

The coordinates of electrons and nuclei are expressed with respect to a frame that moves with the nuclei, so that the nuclei are at rest with respect to this frame. The frame stays parallel to a space-fixed frame. It is an inertial frame because the nuclei are assumed not to be accelerated by external forces or torques. The origin of the frame is arbitrary, it is usually positioned on a central nucleus or in the nuclear center of mass. Sometimes it is stated that the nuclei are "at rest in a space-fixed frame". This statement implies that the nuclei are viewed as classical particles, because a quantum mechanical particle cannot be at rest. (It would mean that it had simultaneously zero momentum and well-defined position, which contradicts Heisenberg's uncertainty principle).

Since the nuclear positions are constants, the electronic kinetic energy operator is invariant under translation over any nuclear vector. The Coulomb potential, depending on difference vectors, is invariant as well. In the description of atomic orbitals and the computation of integrals over atomic orbitals this invariance is used by equipping all atoms in the molecule with their own localized frames parallel to the space-fixed frame.

As explained in the article on the Born–Oppenheimer approximation, a sufficient number of solutions of the Schrödinger equation of leads to a potential energy surface (PES) . It is assumed that the functional dependence of V on its coordinates is such that

for

where t and s are arbitrary vectors and Δφ is an infinitesimal angle, Δφ >> Δφ2. This invariance condition on the PES is automatically fulfilled when the PES is expressed in terms of differences of, and angles between, the R_i, which is usually the case.