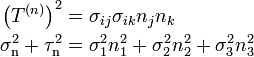Mohr's Circle - Mohr's Circle For A General Three-dimensional State of Stresses

Mohr's Circle For A General Three-dimensional State of Stresses

To construct the Mohr's circle for a general three-dimensional case of stresses at a point, the values of the principal stresses and their principal directions must be first evaluated.

Considering the principal axes as the coordinate system, instead of the general, coordinate system, and assuming that, then the normal and shear components of the stress vector, for a given plane with unit vector, satisfy the following equations

\begin{align} \left( T^{(n)} \right)^2 &= \sigma_{ij}\sigma_{ik}n_jn_k \\ \sigma_\mathrm{n}^2 + \tau_\mathrm{n}^2 &= \sigma_1^2 n_1^2 + \sigma_2^2 n_2^2 + \sigma_3^2 n_3^2 \end{align}\,\!

Knowing that, we can solve for, using the Gauss elimination method which yields

\begin{align} n_1^2 &= \frac{\tau_\mathrm{n}^2+(\sigma_\mathrm{n} - \sigma_2)(\sigma_\mathrm{n} - \sigma_3)}{(\sigma_1 - \sigma_2)(\sigma_1 - \sigma_3)} \ge 0\\ n_2^2 &= \frac{\tau_\mathrm{n}^2+(\sigma_\mathrm{n} - \sigma_3)(\sigma_\mathrm{n} - \sigma_1)}{(\sigma_2 - \sigma_3)(\sigma_2 - \sigma_1)} \ge 0\\ n_3^2 &= \frac{\tau_\mathrm{n}^2+(\sigma_\mathrm{n} - \sigma_1)(\sigma_\mathrm{n} - \sigma_2)}{(\sigma_3 - \sigma_1)(\sigma_3 - \sigma_2)} \ge 0 \end{align}\,\!

Since, and is non-negative, the numerators from the these equations satisfy

as the denominator and
as the denominator and
as the denominator and

These expressions can be rewritten as

\begin{align} \tau_\mathrm{n}^2 + \left^2 \ge \left( \tfrac{1}{2}(\sigma_2 - \sigma_3) \right)^2 \\ \tau_\mathrm{n}^2 + \left^2 \le \left( \tfrac{1}{2}(\sigma_1 - \sigma_3) \right)^2 \\ \tau_\mathrm{n}^2 + \left^2 \ge \left( \tfrac{1}{2}(\sigma_1 - \sigma_2) \right)^2 \\ \end{align}\,\!

which are the equations of the three Mohr's circles for stress, and, with radii, and, and their centres with coordinates, respectively.

These equations for the Mohr's circles show that all admissible stress points lie on these circles or within the shaded area enclosed by them (see Figure 3). Stress points satisfying the equation for circle lie on, or outside circle . Stress points satisfying the equation for circle lie on, or inside circle . And finally, stress points satisfying the equation for circle lie on, or outside circle .

Read more about this topic:  Mohr's Circle

Famous quotes containing the words circle, general, state and/or stresses:

    “Change begets change. Nothing propagates so fast. If a man habituated to a narrow circle of cares and pleasures, out of which he seldom travels, step beyond it, though for never so brief a space, his departure from the monotonous scene on which he has been an actor of importance would seem to be the signal for instant confusion.... The mine which Time has slowly dug beneath familiar objects is sprung in an instant; and what was rock before, becomes but sand and dust.”
    —Charles Dickens (1812–1870)

    “What use Milton, a silly story
    Of our lost general parents,
    eaters of fruit?”
    —Gary Snyder (b. 1930)

    “The longer a woman remains single, the more apprehensive she will be of entering into the state of wedlock. At seventeen or eighteen, a girl will plunge into it, sometimes without either fear or wit; at twenty, she will begin to think; at twenty-four, will weigh and discriminate; at twenty-eight, will be afraid of venturing; at thirty, will turn about, and look down the hill she has ascended, and sometimes rejoice, sometimes repent, that she has gained that summit sola.”
    —Samuel Richardson (1689–1761)

    “American families, however, without exception, experience a double message in our society, one that claims a commitment to families and stresses the importance of raising bright, stable, productive citizens, yet remains so bound by an ideal of “rugged individualism” that parents receive little support in their task from the public or private sectors.”
    —Bernice Weissbourd (20th century)